Inhomogeneous Deformation

The Basics

Let's start where I left off a long time ago: the basic stress - strain curve in a tensile test. Here is the old figure plus the relevant text:


Tensile stress - strain diagram of some steel

"The machine indicates the stress and strain values for the ultimate tensile strength and fracture by blue lines. It can do that because it is smart enough to find the maximum of a curve and the end of one.
It does not indicate the critical yield strength R_P because it is not smart enough to detect that. In fact, there are special definitions and recipes to arrive at a number for R_P but I will not go into that.
In the curve shown the location of R_P is plain to see but that is not always so. We also see that some strange things go on for a while after R_P was reached. The curve gets kind of wobbly, and much is made of this in real testing.
It is not unusual that the stress - strain curve wiggles a bit or runs through a small peak as soon as plastic deformation begins at R_P. That just signifies that before plastic deformation can proceed in a major way by dislocation movement, the dislocations either have to be generated first, or ripped off from the obstacles that pinned them down, or both. That happens at somewhat different stresses in different parts of the sample, and that's why the curve might become "noisy". It's an important phenomena and a nuisance. Since at this point we do not yet know what dislocations are, I will get back to this point much later"

Now it is much later. What, exactly, is going on in the wiggly part (also known as yield point elongation) of the stress - strain curve shown above for some steel?
The answer is: the basics are clear, the details get messy.

The first thing to note is that we have two independent but nonetheless often interconnected mechanisms at work here.

The stress required to "make" dislocations is typically higher than the stress required to move them.
A dislocation in a "dirty" material might be surrounded by a "cloud" of dirt atoms. That means that the concentration of dirt atoms is larger in the vicinity of the dislocation line than in in the bulk of the material. That makes dislocation movement in the cloud more difficult in comparison to moving a dislocation in regular parts of a crystal.

To see what the first effect can do, we only need to perform a tensile test with a material that contains no dislocations (or other defects) whatsoever. Such a material exists; it's called a "dislocation free single crystal of silicon" (Si), the basic stuff for making microelectronic components. And no, silicon is not brittle - provided you heat it to temperatures above about 700 ^oC (1292 ^oF). The figure below shows schematically but close to reality what you will get; I have used a similar figure before:


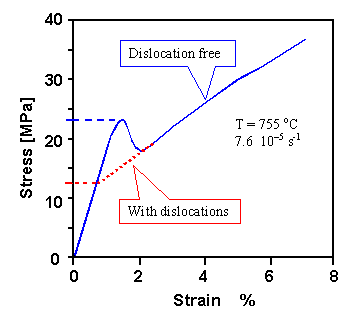Plastic deformation of silicon
Source: Based on the data in: A. Fisher et al.: "Upper yield point of large diameter silicon", Microelectronic Eng., 56 (2001) 117.

Obviously and understandably you need far more stress to start plastic deformation if there are no dislocations. You first have to make some, then they need to multiply. Only if there are enough dislocations around, their movement and not their density will control how much stress you need to produce the required deformation.
The blue line denotes the "upper yield strength" which we can take to be a measure for the stress needed to produce and multiply dislocations.

Now you repeat the experiment with silicon that contains a sizeable density of dislocations - you might just take the strained specimen from your first experiment. What you will get now is the red curve - and a lower yield strength that only measures what it takes to move dislocations.

In the curves up there I have indicated the yield strength by just drawing in some lines "by eye". That is often good enough. However, stress-strain curves without clear switch-over from elastic to plastic deformation do not show an easily seen yield point. Precision-minded engineers therefore came up with a special definition of how to determine yield strength:

Yield strength or proof stress is the stress needed for a permanent strain of 0.2 % (sometimes 0.1 %)

It is often indicated in the notation. For example, R_p0.2 = 100 MPa means that we are dealing with a proof stress of 100 MPa to achieve a 0,2 % permanent deformation .
That answer the first point left open above. The proof stress is by necessity a bit larger than the stress needed to move dislocations, but in contrast to the "true" yield strength it is easy to measure.

So far, so easy. Now it get's a bit more confusing: You can get a similar effect - a peak in the stress-strain curve around the yield strength - in materials where you already have a lot of dislocations loitering around, waiting for action. If they happen to be tied down by a cloud of solute atoms, it will it take some extra force or stress to get them out of what we now will call a " Cotrell - Bilby cloud". As soon as they have managed to escape, they need less stress to move about rather quickly and the solute atoms can not keep pace. Moreover, the applied stress can now decrease without compromising the deformation.
It's a bit like a rock star surrounded by groupies. Most of them are not permanently attached at the closest possible distance but swarm around in the neighbourhood. They certainly impede his movement. Only if he can move faster than they can (he has a Ferrari, after all), he can get away and move around easily again.

It is clear that dislocation imprisonment in a Cotrell-Bilby cloud cannot happen in a clean crystal. You need some ~~sluts~~ dirt that can form a cloud around a dislocation. Not just any dirt but "groupie" atoms that are:

Atomically dissolved.
Happier in the vicinity of a dislocation than in the bulk of the crystal.
Able to impede dislocation movement. That means they should be good solution strengtheners.
Able to diffuse to the dislocations at low temperatures.

The first and second condition is met by many impurity atoms. The third condition actually automatically ensures that the second condition is met as well. If single impurity atoms impede dislocation movement, they do so because they interact strongly with the strain field of the dislocations, and that is just another way of saying that they gain energy by being close to the dislocation line. The fourth condition removes slow diffusors, and that means pretty much all substitutional impurity atoms in iron. At high temperatures, when they are mobile, they can make it to a dislocation but won't stay there very long because the large thermal energy kicks them around vigorously. Only impurity atoms that still can migrate some distance at low temperatures, when they will not get re-emmissioned from a dislocation very quickly again, will do the job.
That leaves us essentially with carbon and nitrogen in iron, just look at the relevant diffusion constants.

Working with Cotrell cloud effects is going to be a bit tricky. If you cool down slowly, pretty much all the carbon will be tied up in cementite and not enough is left to form Cotrell clouds. If you cool down very quickly, some carbon will still be around in supersaturation in the ferrite but may not have had enough time to make it to the dislocations. If you don't have plain carbon steel but alloyed all kinds of other elements, there might now be a competition for the carbon between the "carbide forming elements" and the dislocations, and it's not so easy to predict exactly what will happen.

"And why should we care?", you might ask. Well - for a number of reasons. There are good reasons why such a thing as "interstitial-free steel" is important for the car industry - no Cotrell clouds and thus no annoying "Lüders bands", see below. Contrariwise, the "bake hardening steels", also quite important for the car industry, rely on the formation of Cotrell-Bilby clouds and thus need to have carbon interstitials around.
So there are good reason why we should care.

It remains to see how all this ties in with the topic of this module, which was, as you probably forgot by now, is "Inhomogeneous Deformation".

Lüders Bands

When we subject a normal piece of metal to a tensile test, we typically have a poly crystal containing some alloying elements. Plastic deformation starts as soon as the shear stress on possible dislocation glide planes exceeds the stress needed for moving dislocations on those planes. It is important to realize that the highest shear stress in a tensile test always occurs at an angle of 45^o to the pulling direction.
The normal stress on the specimen is supplied by the machine and is nominally constant everywhere in the specimen because its diameter is constant. The shear stress on all planes inclined with the same angle to the pulling direction is thus the same, and we might expect that yielding, and thus dislocation movement, should start everywhere at the same time. Well - it doesn't!

First of all, possible glide planes in some grains are closer to the 45^o condition than those in other grains. Dislocations in the favored grains thus start to move before those in other grains can do so. Moreover, some of the favored grains contain larger densities of dislocations than others and thus deform more easily that others.

As you see there are good reason why plastic deformation should not start everywhere at the same time but just in some special grains.
That's actually what will happen - the only question is: will you notice it? The answer, as ever so often, is simple: it depends! It depends if you have or do not have a Cotrell-Bilby cloud around your dislocations.

If you do not have a Cotrell-Bilby cloud, you will not notice. Your specimen deforms homogeneously even so it started inhomogeneously at the very beginning. It is easy to see why. As soon as the first dislocations move in favored grains, they start to interact, bump against obstacles like grain boundaries and so on - local work hardening takes place. That means that the stress supplied by the machine will no longer be sufficient to move the "early" dislocations, and the machine now must increase the stress ever so slightly to start dislocation movement somewhere else. And so on. Your stress-strain curve smoothly changes from purely elastic, to dislocations moving in some grains, to dislocations moving in many grains, to fully plastic deformation with dislocations moving everywhere.

If you do have a Cotrell-Bilby cloud, things are completely different. You will notice that plastic deformation does not start everywhere at the same time. As the stress goes up, some dislocations in some favorably oriented grains etc., will rip free of their Cotrell-Bilby cloud prison as soon as the upper yield strength is reached. After that they are free to move easily and a burst of plastic straining takes place in a more or less narrow region or band. That means that deformation in the affected parts is now very easy and the machine must quickly reduce the stress to the lower yield strength because it must keep the strain rate constant.
Only after the first bunch of "freed" dislocations gets stuck again, the stress must go up a little bit again. That frees dislocations in neighboring parts for two reason:

The stress is larger there because the regions deformed in the first step are a now a little bit reduced in cross-sectional area and stress is force divided by area.
The deformation process produced a high density of dislocations.

So a second deformation band develops, mostly right next to the first one. If that happens the stress can go down again. And so on.
In total, the stress will jitter up and down in a more or less stochastic way around the lower yield strength - exactly as you see it in the in the figure above. This happens until all parts of the specimen are finally deforming.

In other words: Deformation at first will be confined to a more or less small region where conditions are "just right". It will progress at about 45^o to the axis of the specimen. The "just right" conditions are likely to start at the "fillet" of the specimen (you won't be able to pronounce the more precise German word "Fußausrundungsfläche" for that), the region where the standardized specimens start to increase their diameter, because the changing radii of curvature provide for some stress concentration.
All in all, deformation proceeds - schematically - like this:


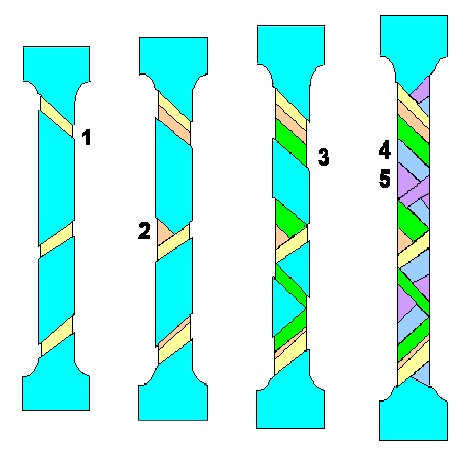Formation of Lüders bands.

Shown here is a more complex case where the first deformation (No. 1) starts at three places. Plastic deformation first occurs in more or less narrow regions, roughly under 45^o to the acting external stress. The specimen gets a bit thinner in these regions (exaggerated above), and this is readily observed in-situ by the appearance of what is called "Lüders bands".
A soon as work hardening in the Lüders bands demands for more stress, adjacent regions (2 ® 3 ® 4 ® 5) become active for the reasons given above.. The process repeats at stress levels that fluctuate for while around a constant value until the whole specimen is deforming. After that, the stress-strain curve will go up as usual, since there are no more dislocations that can be easily moved after "liberation" from their Cotrell-Bilby clouds.

Note that Lüders bands are not the same thing as glide steps observed in single crystals like this old example. Glide steps result on the surface of a crystal because some dislocation source sends a lot of dislocations down the same plane, producing a big step at the surface where they all emerge. Of course, what you see when you look at Lüders bands, are the glide steps at the surface of the last grain. These steps are produced by the dislocations that took up the deformation that runs across the whole poly crystal from the neighboring grain. So there is some relation between glide steps and Lüders bands but the reasons for their formation are entirely different.

Now we understand in principle why we can have inhomogeneous deformation or, expressed in different words, observe the formation of Lüders bands in steel.
Of course, Lüders band formation poses a major problem in metallurgy. When you plastically deform a metal, e.g. to make a fender or some other car body part, by "drawing" a metal sheet into a form, the formerly smooth surface is now speckled and roughened by Lüders band formation, and that is obviously not good. "Orange peel" skin, dreaded by countless females of a certain age, is also known and dreaded by mostly male engineers in the steel industry with regard to the appearance of the the precious "skin" of their drawn parts. So it is the avoidance of Lüders bands that keeps us rather busy. It sure helps to understand the mechanisms!

Lüders bands have been observed and described rather early, long before Cottrell and Bilby supplied the explanation for their occurrence in 1950. To be fair, I should say that it was actually G. Piobert, a French General, who first briefly mentioned in 1842 some markings or patterns that were probably Lüders bands, and that he observed on metal that was deformed by shooting at it with artillery. But it was W. Lüders who clearly described and illustrated the bands appearing on the surface of polished metal upon deformation in 1860 (of course only the outward appearance and not the mechanism), and that's why we call them Lüders bands. Don't feel sorry for the French guy for loosing out in naming the effect. He had, after all, far more fun in doing the experiments.

Strain Ageing

Cotrell-Bilby clouds do not only produce Lüders bands, they are also responsible for an effect called strain-aging. Imagine a deformation experiment where you have deformed your specimen to some extent. Release the load for a short time, and then continue. Normally, the stress-strain curve will continue just as it would have if you wouldn't have taken a break.

However, if your material is given to strain-aging, and your break was sufficiently long, the material might now be harder and less ductile. This is schematically shown below in a figure taken right from one we had before.


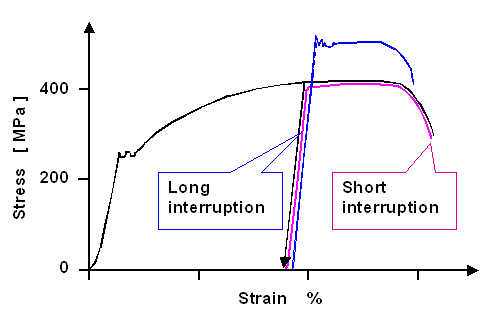Strain ageing in C15 steel

What happens is clear. When you stop deforming, your dislocations stop moving and just stay where they are. They will find themselves in a normal part of the crystals that does not contain more than its fair share of interstitials. When you re-apply the load within a short time, they move exactly as before.
However, if you allow enough time for the dislocations groupies to catch up with their prey, Cotrell-Bilby clouds will form around the resting dislocations. It is now more difficult to move again compared to when they stopped moving at the end of the first deformation.

And now you have a problem and an opportunity:

First the problem:
The steel sheet you buy for making your car parts or whatever, often has been plastically deformed before you get it; for example because that gave it the shape it has. How that sheet is going to deform when you are ready to use it then depends on how much strain-ageing might have been going on while it was sitting on your "shelf". I actually gave you an example for that without mentioning the term "strain-ageing" there.
Obviously, that's not good. You must find ways to get around this problem. Now, if you can avoid interstitials, you will not have all these Lüders band and age-hardening effect. So go and buy yourself so-called IF steel, "interstitial-free steel", or some other grades of steel that do not show strain-ageing effects.

Now for the opportunity:
If you know exactly what you are doing, you buy an optimized steel for what you have in mind. You first deform your relatively soft steel into the shape you want, and then you allow strain-ageing to happen, making it considerably harder after you shaped it. Of course, you can speed up the process of Cotrell-Bilby cloud formation by increasing the temperature somewhat. If you are smart, you use a heating process for that you need to do anyway for independent reasons - like the curing of the paint you put on body parts.
Now we are talking BH or bake-hardening steels. Another kind of steel that makes big inroads into the car industry at present.

To illustrate these points I give you the typical stress-strain or deformation curves for three modern steels; all with a nominal yield strength of 350 MPa.


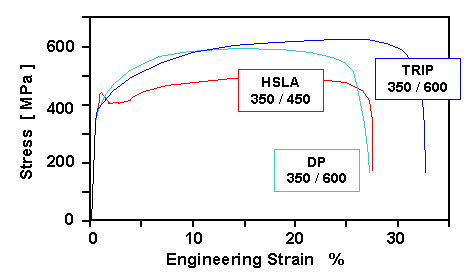Comparison of stress-strain curves for three steels with the same nominal yield strength

The not-quite-so-modern high-strength low-alloy (HSLA) steel does show the typical peak around the yield strength and thus must be expected to incur Lüders bands and strain-ageing if you're not careful.
The dual phase (DP) steel and the transformation-induced plasticity (TRIP) steel do not show this. They are also more ductile and have a higher tensile strength (nominally 600 MPa vs 400 MPa) than the HSLA steel.
The picture also serves to illustrate two points:

Property differences that might look rather small to a lay person might be rather decisive for applications.
Hardness (about the same thing as yield strength) doesn't tell you all that much. All three steels here have the same hardness!

You find more to those steels in the links given above. Here I just wanted to give you a taste treat for what is going on with the Science and Engineering of steel since about 1980.