| |
Preface |
| |
This module draws heavily on stuff dealt with in the next
two chapters. Nevertheless it belongs into this chapter. See it as a taste treat of a science module and look at it again
after reading the next two chapters. |
| | |
|
|
| |
Energy Considerations |
 |
Calculating exactly why, when and how some material fractures is about the most
difficult undertaking you can get involved with as a Material Scientist and Engineer. Since I never did that, and since
the issue is quite complex, I can only look at some very basic principles here.
First, we consider the basic dogma of
fracture mechanics: |
|
| |
| |
Fracture occurs when cracks propagate.
The cracks might have been there from
the beginning or are formed under load.
|
|
| |
|
|
 |
This figure illustrates the basic idea: |
| |
|
|
|
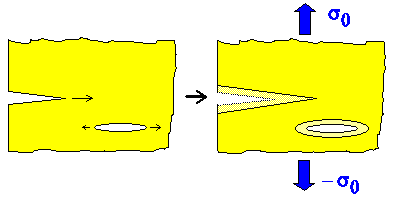 |
| Small ("nano") cracks at the surface and inside some material. |
The cracks grow under load, leading to fracture. |
- Fracture occurs if cracks grow and get larger.
- Cracks get larger if the crack tips move as schematically indicated.
- Crack tips move if the tensile stress s0 applied to the specimen (blue
arrows) is larger than some critical stress scrit
|
|
| |
| |
|
 |
Fracture occurs because unavoidable microcracks
(better: nanocracks) that are always present at the specimen surface or inside
the specimen, start to grow if the stress at the crack tip reaches a critical value.
If cracks grow, they can do that
very fast, roughly with the speed of sound. In the example above, where the specimen is under tensile stress, the crack
tips would move roughly perpendicular to the applied stress direction. As soon as the crack tips start to move, fracture
would be almost instantaneous - except if the conditions at the crack tip change, e.g. because the crack tip hits an obstacle
and either stops or gets deflected. |
 |
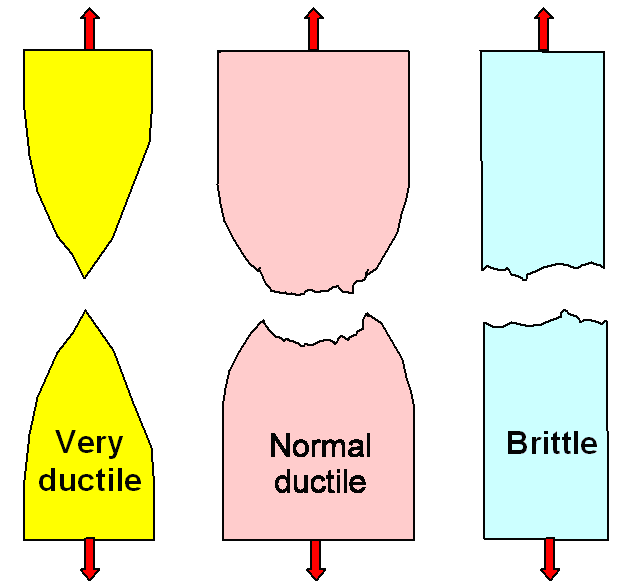
So far this holds for brittle and ductile
specimen. But of course there are major differences between the two. Ductile materials only fracture in a tensile test after
extensive plastic deformation took place; look once more at the typical
stress-strain curves and the figure below for the two extreme cases to appreciate that. |
| |
|
|
|
 |
Fracture occurs because cracks grow if the
stress is sufficiently high |
|
| |
| |
|
 |
Note that as far as fracture is concerned, there is a big and obvious difference between tensile and compressive stress, in contrast to just plastic deformation. Tensile stress opens the crack, compressive stress closes
it. That's why concrete or most "stones" can take enormous compressive stresses, e.g. at the base of Khufu's (Cheops)
pyramid, but fail quickly under tensile loads. The solution to this problem is re-enforcing you concrete with steel that
can take the tensile stress. |
 |
Let's first look at a quite general fracture model. We ask ourselves why crack tips do not start running already at low stresses. Why do specimen as shown above do
not break immediately, if stressed? That is not a simple question.
So let's look at the most simple (haha) case first:
Let's fracture an ideal perfect brittle
crystal with no nanocracks and so on. We know that we can fracture anything, even an ideal crystal, because
the First Law of Materials Science always
applies. |
|
 |
The spring model of crystals,
where you consider the bonds between the atoms to behave like little springs, gives an easy-to-calculate first answer. Well,
not quite that easy but not too difficult with a few well-chosen approximations. What we do is to simply calculate the total
work or energy needed to pull out the bond-springs between the atoms to a large distance,
effectively fracturing the crystal. In the link above that's done with a lot of math, but the result
doesn't get us very far. The reason is that in this model we fracture all the bonds
in one fell swoop, whereas in reality we fracture them "one-by-one" as a crack grows - and that is considerably
easier.
The decisive insight comes from asking a simple question: |
| | |
| |
A lot of energy is needed to fracture
a material.
Where does this energy go?
|
|
| |
|
|
 |
You have invested a lot of work for destroying a piece of crystal by ripping
it apart. Where is that energy now? Energy, after all, is conserved.
It cannot simply disappear.
One might guess that the fracture energy will turn to heat, making the two fractured parts
somewhat hotter. It does that, allright, but that is only a minor side effect. Most of the energy, as Alan Arnold Griffith first realized, is needed to create the two new surfaces that did not exist before fracture.
Surfaces, or more generally interfaces,
possess some surface energy of their own.
Atoms in an interface like the surface are not as happy as atoms in the interior of a perfect crystal, where they have exactly
the environment they like best. That means that this environment has the smallest
(free) energy by necessity. By default, any environment different from that of a perfect crystal must have a higher
energy. Chapter 4 and chapter 5 deal with this in detail. |
 |
OK - enough foreplay now, time to start the serious stuff.
If we calculate the energy needed to rip some of the bonds of a perfect crystal apart, and equate that with the energy needed
to generate the new surface, we obtain a rather simple (approximate) equation: |
| |
|
|
|
|
scrit(ideal) » |
æ
ç
è |
Y · g
a0 | ö
÷
ø |
1/2 |
|
|
| | |
|
|
 |
We already know the meaning of most symbols, the rest are easy:
- scrit is, of course, the critical or maximum stress one can apply. Go a
smidgen above scrit , and instantaneous fracture occurs.
- Y, as always, is Young's modulus
and thus (as we are going to learn soon) a measure of the "strength"
of the bonding springs.
- a0 is simply the distance between the atoms, roughly around 0.3 nm.
- g
is the specific energy of the surface (measured, e.g., in J/m2) and thus a quantity that can be measured
or calculated.
|
|
 |
The meaning of the equation is just as easy to see:
- For the materials under consideration, get the three numbers for the three quantities on the right hand side. That is
not difficult, most of that stuff is known and recorded somewhere.
- Multiply Y and g, divide by a0, take the square
root - and you know the maximum stress your brittle material can take without fracturing.
|
|
 |
Or do you? No, you don't. Any material you
like to test will fracture at much smaller stresses than the one calculated above. Not
just a little bit smaller, but 10 or 100 times smaller!
Worse, the fracture stresses measured
for nominally identical materials, while always much smaller than the calculated one, are often wildly different.
|
| |
|
|
|
|
| |
|
 |
Without reliable numbers for the stress a material can take, there is no reliable
design or construction of mechanical machines. True, we do not built airplanes and so one from brittle materials, but the
situation for ductile stuff like aluminum alloys is not much better as we shall see.
And yes, the problem has been solved throughout the millennia by simply making the mechanically challenged parts of
a construction far bigger than absolutely necessary. If your new temple collapsed because the columns (brittle material!)
fractured under the weight, you made them bigger and bigger. Same thing for early metal
constructions: they were rather solid and not given to fracture. Later metal constructions
like early railway stuff and steam boilers fractured like crazy, killing lots of people. So good steel was invented and
heavy parts were made. Problem solved once more. Well, yes, but those things wouldn't fly
- far too heavy. |
|
 |
There is a need to make light-weight constructions that can fly, go very fast
(like non-American trains), do not break apart in cold water (like some American ships),
or get very high mileage (like non-American cars). If you want to do this, you depend rcompletely on reliable
numbers for what the material can take - in terms of critical loads and many other parameters. |
 |
The reason for premature fracture, as Griffith
first realized, are tiny defects in the materials, in particular something Griffith called microcracks
and that I will call nanocracks, because it causes already sufficient trouble if those
defects are in the nanometer range. Nanocracks (and other defects) are always present in real
materials. Griffith didn't just realize the relation between fracture and defects, he performed interesting calculations,
getting extremely important results. |
|
 |
To get a taste treat of what Griffith did, let's now look at a sample under tension
as above, but with a nanocrack as shown below in some more detail.
The first thing to realize is that a roughly circular
area (in three dimensions it would be a cylinder) around the nanocrack (darker blue) is more or less stress-free. Just imagine
the light blue part below to be a rubber band with a slot and you see what I mean. Since "nobody" pulls at the
already fractured part, there cannot be much stress there. |
|
| |
| |
 | Stress situation around a microcrack
|
|
| | |
|
|
 |
Very roughly, the area inside the blue circle with a radius d =
half width of the crack is stress free since "nobody" pulls at the atoms at the surface of the crack. In reality,
the stress increases gradually from zero at the crack surface to the bulk value with increasing distance from the crack.
Maximum stress occurs at the edges of the nanocrack. The stress state there is not uniaxial
as in the bulk of the material but more complicated. Whatever, rather similar energy
arguments as for fracturing an ideal crystal apply:
- Growth of the nanocrack generates new surface and that "costs" surface energy g.
- Growth of the nanocrack generates a larger stress-free region and that releases or "pays" elastic energy -
fewer bonding-springs must be elongated.
Crack growth thus is only a good energy investment for a crystal if more elastic energy is released than needed for
increasing the surface. The equation resulting from working that out is called Griffith
criterion; here it is: |
| |
| |
| |
| s crit(real) |
» |
æ
ç
è |
2Y · g
pd |
ö
÷
ø |
1/2 |
|
|
| | |
|
|
 |
This is pretty much the same (approximate) equation we had for the perfect ideal
crystal right above, except that the distance a0
between the atoms is replaced by the half-width d or about the size (see the figure) of the nanocrack! And
never mind the (2/p )½ = 0.8; it just appears because I need it later.
All this is approximate, anyway, and a factor of 0.8 is close enough to 1 to be of no importance here. |
|
 |
What that means is obvious. A crystal containing just one
nanocrack with a width of, for example, 2d = 60 nm (or roughly 25a
0), will fracture at roughly 1/5 of the stress needed to fracture an absolutely perfect crystal.
Consider that a 60 nm nanocrack is far too small to be seen with the best optical
microscopes, and that a surface containing nanocracks of this size would still look
well polished. The message is clear: |
| |
| |
| |
Extremely small nanocracks (= defects in
a (brittle) crystal) will dramatically
reduce the fracture strength.
|
|
| | |
|
|
Stress Considerations |
 |
Looking at the total energy of a large system is always a good thing to do. It
will tell what the system as a whole is apt to do. It's like looking at national economics. The guys looking into this will
tell us how the economy of the country could benefit if some extra money would float around and we all do the right thing
with it. On the other hand, if your wife will come into possession of some extra money, chances are that she will neither
know nor care about what would be good for the national economy, but dispose of the windfall in any way she likes. |
|
 |
Nanocracks are no better than your wife you. They neither know nor care
about what would be good for the total energy of the crystal. All they
know is that in order to move, they must break the bonds between the atoms right at the crack tip. The figure below
illustrates that. |
|
| |
| |
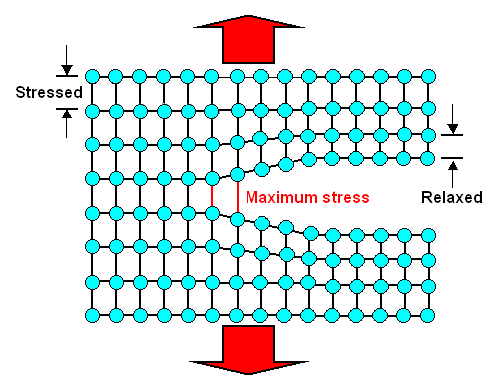 |
| Maximum strain / stress occurs at the crack tip. |
|
| |
| |
| |
 |
Shown is strain. The larger the distance between atoms
on top of the equilibrium distance, the larger the strain. Since the strain is proportional to the stress, it is essentially
the same thing. |
 |
As soon as the stress at the crack tip is large enough to rip apart the bonds
between the atoms there, the crack tip will move. The crack gets larger and the crystal fractures. No atom there gives a
damn about surface energies, etc.
What we must do now is to calculate that stress. |
|
 |
That's not an easy job since the stress at the crack tip is not uniaxial as in the bulk of
the crystal but more complex. Nevertheless, in a good approximation, the stress
stip at the crack tip for a uniaxial
situation as shown can be calculated to |
| | |
|
| | |
| stip |
» 2s0 |
æ
ç
è |
d
r |
ö
÷
ø |
1/2 |
|
|
| |
|
|
 |
s0 is simply the nominal tensile stress
acting on the specimen; d is the half-width of the crack as before, and r is the radius of a
sphere that one could inscribe at the crack tip and that measures how acute or blunt the crack tip is. This is illustrated
below. |
|
| |
| |
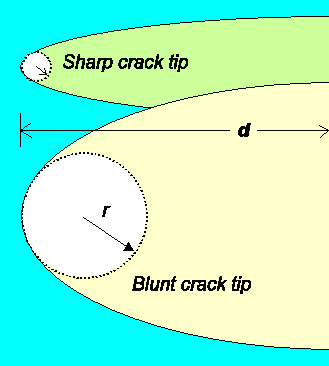 |
Describing the sharpness of the crack tip by inscribing
(approximately) a circle with radius r . |
|
| | |
|
|
 |
In any case, the stress at the crack tip is larger than in the bulk of the material,
an effect called stress concentration. At a sharp tip (small radius r)
the stress is larger than at a blunt tip (large radius r). The crack thus will start moving at lower loads
than at the blunt tip. |
|
 |
Is that contradicting the earlier energy consideration? Not at all. Like in national
economy, the things that are predicted on the large scale come about without direct consideration of what is going on at
the small or individual scale. If we look at the small scale (atoms) or large scale (energy of zillions of atoms) is simply
a matter of convenience. |
 |
We have almost all the major ingredients now to understand the basic principles
of fracture in brittle and ductile materials. What's missing is the basic principle
behind ductility. |
|
 |
In other words: What happens if plastic deformation
occurs? In yet other words: What other kinds of defects besides nanocracks do we have in crystals and how is all of that
connected? We must know a bit about that before we can go on. |
|
 |
So read chapter 4 and
chapter 5 of the backbone now. |
| | |
|
|
|
|
| |
|
© H. Föll (Iron, Steel and Swords script)




