Fracture Mechanics II

Flashback and new Goals

If you have read through the backbone of this chapter, you are now a better person because you know something about plastic deformation, dislocations, and all the other defects making crystals more colorful in both meanings of the word. That's good because now I can continue the "Fracture Mechanics" stuff I have started when you were an ignorant....(insert you job title or whatever else is right).
Let's quickly review the essentials of the first Fracture Mechanics module. The figure below illustrates the basic ideas:

$Microcracks and fracture$
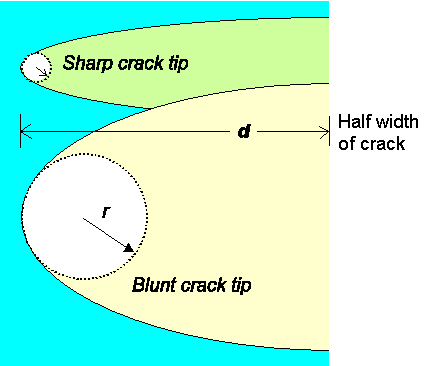Geometry of microcracks and fracture mechanics.

The basic points to recall are:

The rapid growth of pre-existing small " microcracks", characterized by their half-width d and tip radius r, leads to fracture in brittle materials.
On a macroscopic level, the fracture criterion ("Griffith criterion") can be derived from an energy consideration. Fracture in uniaxially strained materials occurs at some critical stress s_crit if more strain energy is released than surface energy generated. The basic equation for this was (with Y = Young's modulus, g = specific surface energy):

s_crit	=	æ ç è	2Y · g pd	ö ÷ ø	1/2

The criterion is purely energetical and does not take into account the crack tip geometry and the stress concentration there.
The criterion is only applicable to perfectly brittle materials under uniaxial stress with cracks oriented perpendicular to the stress direction.

In other words: While Griffith's work was seminal in the sense that it produced major insights into fracture science , it is rather limited and does not allow fracture engineering. One of the reasons is that even for perfectly brittle materials, the surface energy g is not really a good parameter. It is not all that well defined for real materials that have never a clean surface but always a "dirty" one, covered with oxide and God knows what else.
We thus need to modify the simple approach, considering in particular ductility and more complex stress situations than just uniaxal deformation.

Fracture and Ductility

The first thing to do is to rewrite Griffith's criterion to make it more general. We do that by defining two new parameters as follows:

s_crit	=	K_Ic ( pd)^½	with	K_Ic	=	(2Y · g) ^½

K_Ic	=	critical stress intensity factor or fracture toughness (for uniaxial stress)

Formally, the new quantity "critical stress intensity factor" K_Ic would be equal to (2Y · g)^½ . This factor relates to crack growth by giving the energy needed via g , and the energy gained via Y. Let's forget that now and accept that K_Ic somehow takes care of all energies involved instead of just the surface energy g and simple strain energy.
Just as Y and g are material "constants", somehow encoding a major property of a given material, so is K_Ic. It's just no longer simply the product of the two old parameters but something new that we measure since we cannot really calculate it very well.
If we know the critical stress intensity factor of some material, we know at what (uniaxial) stress it will fracture if it contains microcracks with half-width d.

If we now plot the critical stress s_crit versus crack size d we get this curve:

$Brittl fracture curve$
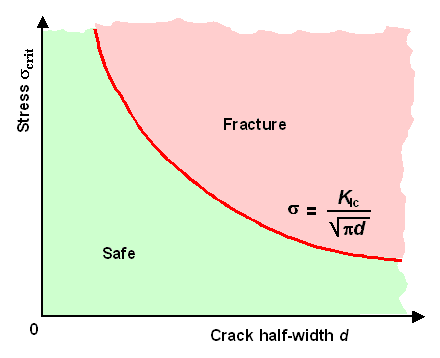Brittle fracture with the stress intensity factor .

The red line, described by the simple equation with the critical stress intensity factor or fracture toughness K_Ic separates safe combinations of crack length and stress from combinations where instantaneous fracture occurs. This is still nothing new but just the Griffith criterion expressed as graph. .

So far I have just renamed things; there is not really something new. The new inputs come now. They are:

The curve separating save and deadly combinations of stress and crack size (the red curve above) can actually be measured in some special test. That's how we get numbers for K_Ic
So far, everything concerned only brittle materials. But we can take care of ductility now. All we have to do is to add a straight line at the specific yield stress s_p of the material to the figure above

What we get is this:


Brittle fracture with the stress intensity factor .

The red line, as before, separates separates safe combinations of crack length and stress from combinations where instantaneous fracture would occur if there would be no plastic deformation. The mauve line gives the yield stress s_p; above it only plastic deformation will occur. That means that nowhere in the material can be stresses higher than the yield stress; they would always be released because dislocations form and move, and thus change the shape of the material in such a way that the stress is reduced.
If we now measure at what kind of combinations of crack width and stress sudden fracture will occur, we get the blue curve. As one would expect, it is a smooth change-over from brittle-like fracture behavior to ductile behavior, where the specimen does not fracture under stress, but just "gets longer".
Of course, the transitions are not precise and sharp. I tried to do justice to that by using wobbly lines and mixed colors at the boundaries.

It is convenient to define a critical crack half-width d_crit as shown, using the intersection point between the two curves for fracture only, or plastic deformation only.

I guess you see the good news yourself: You don't have to worry anymore about small microcracks! Sudden fracture does not occur at all for stresses above the yield stress and cracks smaller than some critical crack half-width d_crit. You will experience yielding before fracture. That might not be great but still far better than the opposite.

For cracks smaller than d_crit, plastic deformation will take care of them. Since, as we learned before, the stress around the crack tip is higher than the global or macroscopic stress, plastic deformation at the crack tip starts long before the crystal deforms as a whole. This blunts the crack tip and reduces the stress to levels where the crack does not propagate.
This is not a minor effect. The critical crack length might increase to huge values like millimeter! Here is a figure showing this with a real example:


Data for cracking an aluminium alloy at –195 ^oC (–320 ^oF) .
Source: Redrawn after T.W. Orange: "Fracture toughness of wide 2014-T6 aluminum sheet at -320 ^oF", NASA TN D-4017, 1977.

This kind of aluminum alloy can live with cracks as long as about 5 mm (!!!) without catastrophical fracturing or fracturing before yielding.
In other words: while you can never outsmart the first law of Materials Science, you much prefer that some pressure vessel, for example, starts bulging and sort of getting bigger because it deforms plastically, before it finally cracks and explodes, to sudden fracturing / explosions without any warning. Same thing for that steel girder. Slow bending because it elongates plastically before it finally breaks, while not good, is still much preferable to sudden rupture. And so on.

Measurements like the one above allow to extract values for K_Ic and for the yield stress s_p. Of course, we know s_p from independent tensile testing experiments already, so we even have a check if everything is as it should be. Here are a few numbers:

Material	Fracture Toughness K_Ic [MPam^½]	Yield Stress s_p [MPa]
Steel (AISI 1144)	66	540
Cr-Mo-V Steel (ASTM A470-8)	60	620
High-strength Steel (18-Ni maraging)	123	1310
Al alloy (7075-T651)	29	505
Ti alloy (Ti-6A1-4V)	66	925
Polymers (Polyester, PVC, PET)	» 0.5 - 5 0.6, 2.4, 5.0	-
Ceramics (Glass, concrete, "china" (Al₂O₃ ))	» 1 - 8 0.76, 1.19, 4.0	-
Source: N.E. Dowling: "Mechanical Behavior of Materials", 3rd edition 2007, Pearson / Prentice Hall

With these numbers we can "somehow" calculate the blue curve and thus the critical crack length d_crit. This means that we now have design criteria; we can start to construct things that do not break. Fracture engineering starts here.

Unfortunately, it doesn't end here. There is far more to making safe things that do not fracture now or ever, provided my kids or my wife don't get to (ab)use it. Topics to consider, for example, are:

Accounting for cases where the specimen dimensions are not far larger than the crack size (always assumed so far). This leads to rather long equations and a lot of tricky approximations.
Accounting for more complex geometries than just uniaxial stress. This leads to more fracture toughness numbers, labeled, for example: K_IIc and K_IIIc ; the "_I" so far always referred to simple uniaxial cases.
Considering the precise stresses and stress distributions at the crack tip, and not just the approximations used so far (that's where the "simple" K comes in). You definitely do not want to know about this.
Calculating K_Ic instead of measuring it. Something to avoid at all costs.
Considering rapid deformation, i.e. building up stresses faster than they can be relaxed by dislocation movements. Fun to do experimentally (e.g. in sword fights or by exploding things), but very hard to calculate.
Considering what could happen fracturewise as time progresses. Keywords are "creep" and "fatigue".
Going for "safe" design, allowing to make parts that do not fracture even if something goes wrong (your wife uses your knife as a screw driver, your car hits a tree, your satellite is hit by a small meteorite, .... ).