| |
12.2.6 Static Properties of Composite Swords |
| |
A Little Bit of Theory: Plastic Deformations of Composite
Swords |
 |
Here we look a bit more closely on how composites perform in static mechanical
testing. First we look at how a composite made from ideal materials performs in a classic
tensile test experiment. This is a good exercise to get a first idea of what we are up to. Next I will generalize to real composite swords made from not-so-ideal materials.
We start with comparing the stress-strain
diagrams of a hard ("red") and soft ("blue") ideal steel. They are shown below. |
| |
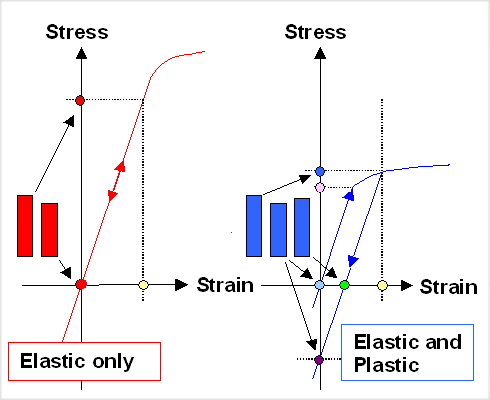 |
Stress - strain diagram for a hard steel, deforming only elastically, and soft steel,
deforming elastically and plastically
The rectangles sybolize shape change |
|
|
 |
We apply stress until a certain elongation (=strain) is reached that shall be
the same for both steels. It is indicated by the yellow dot on both strain axes. The red steel can do this strain at a stress
(red dot on the stress axis) that is still in the purely elastic deformation region. The blue steel deforms plastically
after its yield stress is reached (pink dot at the stress axis; at the deviation from the straight line). It is evident
that the red steel needs more stress (red dot on its stress axis) than than the blue steel (blue dot on its stress axis)
for the strain chosen.
The red or blue rectangles symbolize the specimen lengths at the various points in question.
When we now release the stress, the red steel goes right back to its original length while the blue steel is plastically,
i.e. permanently deformed and stays a bit longer (green dot at its strain axis). If I want to return it to its original
length I have to "push it down", i.e. apply compressive stress as indicated by the purple dot on the negative
stress axis of the blue steel. |
 |
Now let's do a tensile test with a composite of the two steels as schematically
shown in the next figure. Just weld the two pieces together somehow, making an "ideal" weld, of course. Let's
go through what happens step by step. |
|
|
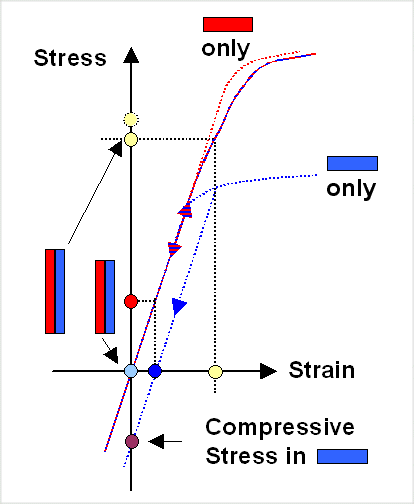 |
| Tensile test for a composite of soft and hard steel |
|
|
- In the beginning of the test both steels are elongated elastically and the stress-strain curve is identical to the first
part of both individual curves above since both steels behave identically - they have the same Young's modulus, after all.
- As soon as the yield stress of the blue steel is reached, it will now deform plastically. That takes less stress than
the elastic deformation, so the total stress the machine needs to apply can go down a bit. Upon further elongation the stress-strain
curve gets a bit flatter and deviates from the straight line as shown.
- The pre-set strain is now reached at a somewhat lower stress level indicated by the lower yellow dot.
- Releasing the stress, the blue steel would like to stay a bit elongated as indicated by the dark blue dot on the strain
axis. That would, however, leave the red steel elastically stretched and "under stress" as indicated by the red dot on the stress axis.
- The red steel is fully attached to the blue steel and acts like a tensed spring. It pulls the blue steel "down",
putting it under compressive stress.
- The final state will be some slight elongation of the red steel, retaining some tensile stress, and some compression
and compressive stress in the blue steel. The "winner" with the lowest stress will be the steel that occupies
a larger volume in the composite.
|
|
 |
In the schematic picture above the relation between red and blue is about 50
: 50 so the effect will be noticeable. The red part will be a bit too long, the blue part a bit too short compared to what
they would like to be at zero stress value. The whole contraption thus would bend somewhat to the left. |
 |
We can immediately transfer these insights to the bending
situation of a one-material sword. As discussed
before, plastic deformation starts on the outside because that's were stresses are highest. In a way, it's not so different
from a composite with a soft and a hard part. Here the outer layer is the soft part. In the beginning the plastically deformed
part of the blade will be just a small part of the whole blade. The plastic deformation effects will be hardly noticeable
in the "stress - strain" curve, which, in the bending case, would be the force vs. deflection curve. You would
not notice the slight bending of the blade after the test because the large part that was only elastically deformed will
have "pulled back" the small plastically deformed parts on the outside.
But the outer layers now contain
a "build-in stress". It is compressive on the side that experienced tensile stress during the experiment and tensile
on the other side.
You may not notice that. However, if you now bend the blade again and in the other
direction (maybe because you suspect that it might no longer be perfectly straight), the outer layers will reach the
yield stress earlier because their built-in stress from the earlier experiment must
be added to the outside stress. Plastic deformation then starts earlier and a larger part of the blade will be affected.
The still far larger part that was only elastically deformed will pull the blade back to being (almost) straight again |
|
 |
You get the point. Repeated bending back-and-forth that appears to be fully elastic
in the beginning, might slowly built up structural changes in your blade that will weaken it. One bending experiment too
much then might damage the blade since it remains bend. Worse, your blade might now fail you in battle when large stresses
are encountered. |
 |
How about bending a composite sword? The katana type (soft inside, hard shell)
can now experience several modes of deformation, e.g.:
- Stresses everywhere are below the yield stresses of both materials. Purely elastic behavior, in other words.
- The yield stress in the outer (hard) layer is reached but no yet in the softer interior
- The yield stress in both materials are reached
- The yield stress in the softer inner materia is reached but not in the outer hard shell.
|
|
 |
his calls for a systematic approach and none better than a good picture: |
| | |
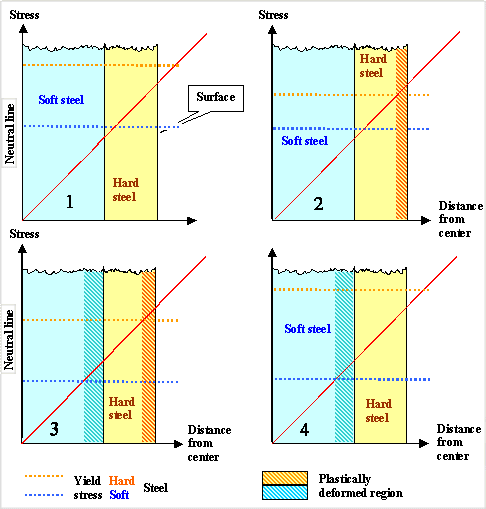 |
| Deformation modes in a composite (soft core, hard outside) blade.
Identical stresses (=fixed bending) is assumed but the yield stresses of the two steels are somewhat different
|
|
|
 |
We look at one half of a section of a blade edge-on. The stress axis, in other
words, would be the edge of the blade and we see the right-hand side of the blade. The soft core (light blue) is somewhat
thicker then the hard outer shell (yellow). The blade is under stress (consider it bend) and the red line shows how the
stress increases from zero at the neutral line smack in the center of the blade to the outside. The blue and red dashed
lines denote the yield stresses of the two steel.
In this example we have the same stress distribution in all four cases
but somewhat different yield stresses of the two steels. What do we see?
Case 1: In both steels the maximum stress is below the yield stress. Only elastic deformation takes place.
Case 2: Stresses are larger then the yield stress in the outer layer of the hard steel but still below the yield
stress of the blue steel. Some plastic deformation in the outer layer occurs.
Case 3: In both steels the yield stress is reached in some parts. Plastic deformation occurs in the outer layer and
deeper inside.
Case 4: Only the soft steel experiences stresses higher than its yield stress. Plastic deformation occurs inside
the blade. |
 |
This gives some impression of what could happen if your two steels are somewhat
different, everything else being the same. Now let's look at what happens to a given blade as the stress is increased. You
just increase the bending to do that as schematically shown by the black curve on top of each diagram. |
| |
|
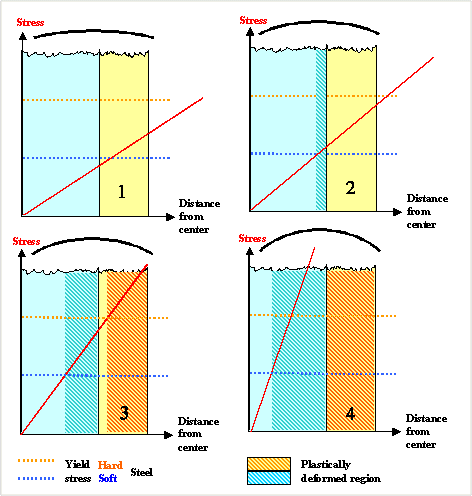 |
| Tensile test for a composite of soft and hard steel |
|
| |
 |
Once more and unavoidably, we start with elastic bending only (case 1) but then
- surprise! - comes plastic deformation of the soft core (case 2) before the outer shell is affected! More bending introduces
plastic deformation in both steels (case 3) and finally we have continuous plastic deformation of most of the body of the
blade (case 4).
In any case, we have more parts of the sword plastically deformed than we would have in a one-steel sword
|
|
 |
It should be clear from what I pointed out before that the only elastically stressed
parts will try to "undo" the plastic deformation of the other parts as soon as the outside stress is relieved.
It should be equally clear that in any case you now have tensile and compressive stresses inside your blade that change
its behavior upon encountering new stress. |
 |
We may draw a first conclusion: We can make the simple composite blade discussed
above from steels with all kinds of yield stresses and in many different geometries. Many different responses to bending
stresses are thus possible for those blades. It should thus be no surprise that some smiths, some schools, some regions,
and so on should have been particularly famous because they happened to produce a particularly good sword. Small details
matter! The precise geometry, the materials used, the way the forging and heat treatments were done, and so on, made the
difference. And I'm still talking ideal materials here! Ulfberht
swords come to mind, for example.
I went through this in some detail to demonstrate a major points |
| |
|
A sword made completely from the
ideal hard steel will have a superior
elastic limit and will always
suffer less plastic deformation
than a composite sword
for a given stress
|
|
| |
 |
I have used the term "elastic
limit" in the sense of how far you could bend a blade before "something happens". It is
a property that is easy to understand in principle but a bit tricky if you look at it in detail. In purely scientific terms
the elastic limit is reached as soon as parts of the blade experience plastic deformation. That means - again in purely
scientific terms - that the elastic limit is nothing but the yield stress of the "softest" component. In practical
terms, however, the situation is more complex for the following reasons:
- As pointed out above, a composite blade made from ideal steel may undergo considerable plastic deformation in parts
but bend back to being almost straight again when the stress is relieved. The "bender" of the sword who does the
test would be inclined to assign an elastic limit to the blade that is well above the one given by the lowest yield stress.
- For a composite blade made form real and inhomogeneous steel, the "scientific" elastic limit is reached as
soon as some particular weak part somewhere in he blade "gives", i.e. deforms plastically. A human tester wouldn't
notice that and once more assign an elastic limit far larger than the "scientific" one.
We have a problem now. A properly measured elastic limit for composite blade tends to be lower than what a human tester
would come up with by just bending the sword. But nobody is interested in the scientific number, firstly because it just
doesn't do justice to a real sword and, secondly, because it almost never exists. Quantitative (and by necessity more or
less destructive tests) are almost never done with real swords. In what follows I will use the term "elastic limit"
therefore in the less precise but more human context. |
 |
If we now look at pattern welded swords, nothing
changes in principle. The major difference is that plastic deformation now will occur here and there in the twisted striped
rods, depending on the local geometry and the resulting stress distribution. |
 |
We now have arrived at a major "theoretical" result for ideal steels: |
| |
The elastic limit behavior of composite
blades is worse than that of the hard
and better than that of the soft steel
Same thing for the plastic behavior,
|
|
| |
 |
"Worse" means that the elastic limit is lower and that more volume
deforms plastically upon bending. |
 |
We have just hit upon what's known as "law of
averages" for composites. It simply states that a specific
property of a composite is some kind of average of the properties of its constituents. Not your usual average, and not necessarily
easy to calculate, mind you. I have given you a detailed example
of how that works for Young's modulus. The consequence is that a composite property can never be outside the range found
by its constituents.
The law of averages is not a real law, just a guideline, though. It is not overly helpful when
it comes to "digital" properties that cannot be easily expressed in numbers. If you combine a ductile and a brittle
material, the plastic deformation or sudden fracture properties cannot be an average of the individual properties . What
numbers would you average? Nevertheless, the composite in question would be less prone to sudden fracture but possibly bend a lot instead.
|
| |
|
| |
Plastic Deformation and Fracture of Composite Swords
Made from Real Old Steel |
 |
If you have worked your way through all of the above, you know that predicting
the precise bending-test behavior of a real
(old) composite sword that consist of different inhomogeneous steels, is well-nigh
impossible. What could happen is sudden fracture after just a bit of elastic bending, major plastic deformation after a
decent elastic interval, or fracture in some parts, plastic deformation in other parts and nothing (i.e. purely elastic
behavior) in the rest.
Whatever kind of real composite blade you have, some regions
will have a lower yield stress than others. Maybe the steel there is of the soft variety, or maybe your hard steel has a
weak spot there. Under loads of all kinds, the yield stress might be reached and plastic deformation occurs, whereas neighboring
regions still are only elastically strained. Without a load, plastically deformed regions will be "pulled back"
to some extent by the elastically deformed regions around them, and the blade looks much the same. But there are now all
kinds of compressive and tensile stresses in the various parts. |
|
 |
That is not necessarilly a bad thing. Real blades may contain considerable internal stresses
from the way they were made. This is particularly true for curved blades with an edge on only one side or even straight
blades with asymmetric cross-sections like backswords.
I gave you a whole (albeit scientific) module on this.
|
 |
However, the biggest problems does not result from variations of the yield stress
in your inhomogeneous blade materials or from internal stress built-up but from the presence of rather large slag inclusions
or other large defects. Around these defects local stresses can be much larger than in the rest of the material, and locally
you may reach fracture conditions even in otherwise ductile steel long before that happens in the undisturbed material.
And as soon as you run into local fracture somewhere inside the material, total
fracture is not too far.
That's particularly true if things happen quickly, like when you get a hit one your blade.
Plastic deformation is the mechanism that distributes the large local impact energy into the volume of the material but
it needs some time to do this. If locally present microcracks opens faster than the energy-dispersing dislocations tied
to plastic deformation can move, it is all over. Your blade will fracture. |
|
 |
Somewhere in all of this lies the explanation why old steel is typically far
more brittle and with a yield strength far smaller than what one would expect from its carbon content. Moreover, you can
expect substantial differences between different batches of the same steel. In a way of speaking, "old" steel
is already a composite material combining "good" parts of the basic material and bad parts. The law of averages
than tells you that old steel is never as good as modern homogeneous steel with the same nominal carbon concentration. |
|
 |
In other words: Looking at composites of old steels is a messy enterprise. Even
the most simple deformation experiment, the tensile test, get's messy. A still quite simple bending test is even messier,
and what happens at a real test - hitting something with your sword - is hard to conceive by theory. Be grateful that it
is not all that difficult to conceive experimentally. |
 |
As far as static mechanical properties are concerned, there seems to be no advantage
for composites of two or more steels. Well - not quite. If one of your steels has extremely bad properties in some parts,
like being too soft or too brittle, "averaging" it with another one, will make this better. Not good, but better.
The brittle part might even fracture - but as long as its just a part of the blade, the blade is now damaged but still serviceable.
I'll look at bit more closely into this in the next sub-chapter. |
 |
If we now consider "real" (old) steel instead of ideal steel, where
does that leave us with respect to our list of swords from above? I'll give you short answers here: |
| 1 |
Bronze sword.
This sword was cast and therefore contains no large "dirt"
particles like slag inclusion and such. It might, however, contain large voids from trapped gas. This has indeed been found
in some old bronze swords and would have resulted in sudden fracture under stress. |
| 2 |
Wrought iron sword
This sword would have less than 0.2 % carbon on
average but would contain slag inclusions and so on, areas with relatively high carbon concentration, and possibly
some phosphorous in some parts. It thus would have been somewhat harder than clean and homogeneous wrought iron. It could
have been relatively ductile or rather brittle, depending on many parameters that are not known. See the net sub-chapter
for examples. |
|
3 |
Good ("eutectoid") steel sword
This would be a very good sword, especially if the edge was quench-hardened - provided the steel is homogeneous. If
it isn't (and that's certainly the case for all old steels), see above. You will find the full range of properties - from
very good to critical since brittle. |
|
4 |
Wootz steel sword
This kind of sword supposedly was very good, if a bit on the
brittle side. However, it is quite likely that wootz blades came in all kinds of qualities. There are very few experimental
tests, one is shown here. The tested wootz steel was not
too bad but generally inferior to modern steel. |
| 5 |
Pattern welded sword
We can be quite sure that swords with a full pattern welded
body were rather lousy; I will get back to this. If they contained a steel core and had pattern welded parts only on the
outside ("veneer" type),
the sword might have been somewhat better. The phosphorous kind of steel always found in pattern welded blades would have
made the sword rather brittle and the bad quality of old steel didn't help much either.
However, cracks that developed
in one part of a "striped rod" might have been arrested in the other one. That damaged the blade but prevented
sudden complete fracture. So pattern welded blades were probably better than really lousy blades made from just one type
of (lousy) steel. They were definitely much showier. |
| 6 |
Japanese type sword (katana) (in its "Viking sword" manifestation)
We
have a hard outside and a soft core, both made from inhomogeneous bad steel, full of slag and other inclusions. However,
at least the steel of real katanas was homogenized by faggoting.
That made not only the distribution of big defects more homogeneous, it also broke them up and instead of a few large ones
you now had a lot of smaller ones. That may have helped to prevent sudden fracture upon impact.
The soft core could
absorb local energy bursts and stop crack growth initiated in the ultra-hard (and completely brittle) edge. "Katanas"
or Viking-type swords may have been the best swords one could
make from lousy bloomery steel but they wouldn't be a match for swords made from modern steel.
If the Viking sword
was made from faggoted steel and if it had a hardened edge has never been thoroughly investigated. Personally, I would be
surprised if the better ones weren't made that way. |
| | |
|
© H. Föll (Iron, Steel and Swords script)



