|
In what follows a few examples for imaging dislocations with TEM are shown.
Where possible, examples have been selected that have been used before (e.g. in the context of dislocation loop formations)
or will be used later (e.g. in the context of defects in boundaries). |
 |
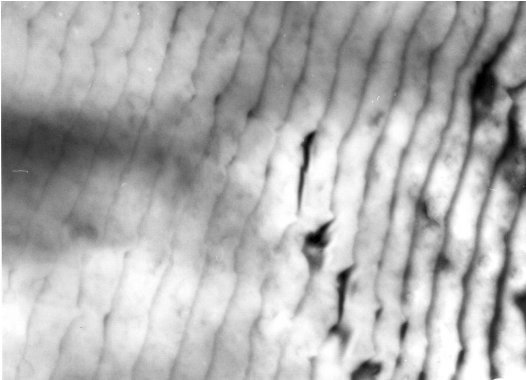
The first example demonstrates the contrast of dislocations as a function of the
excitation error |
| |
|
|
 |
The specimen was bent a little; so the excitation error changes from left to right. On the left hand side,
the excitation error is relatively large; on the right hand side it is small. The contrast on the left is weak, but the
resolution is good; on the right hand side the dislocation appears a as strong, but blurred black line. |
 |
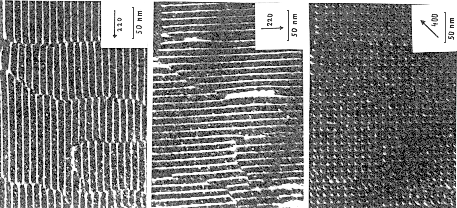
The second example demonstrates the contrast disappearance for g ·
b = 0. |
|
 |
Shown is a network of pure screw dislocations in Si which we will encounter again in the context
of grain boundaries. |
| |
|
|
 |
Only one set of dislocations shows up in the dark field conditions employed for the g =
{220} type of diffraction vector which is parallel to one Burgers vector and perpendicular to the other one. With a
g = {400} diffraction vector both sets of dislocation are imaged, but there is a loss of clarity. |
 |
Next we will see how a dislocation loop can be analyzed. |
|
 |
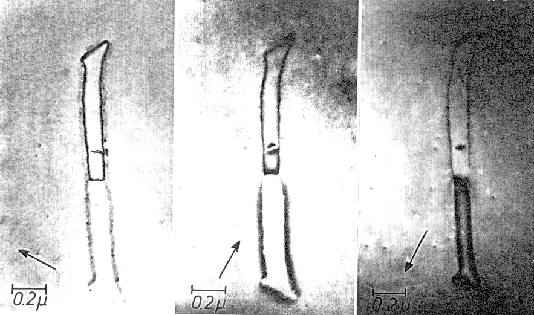
Shown are dislocation loops of the "A-swirl defects"
imaged with two different diffraction vectors (drawn in as arrows) and a +g/–g pair. In the first image, the
contrast of the lower dislocation loop has disappeared (the fuzzy line is due to the precipitates along the dislocation
line). The two pictures on the right show the lower loop, the image is wide or narrow, depending on the sign of the diffraction
vector g. |
| |
|
|
 |
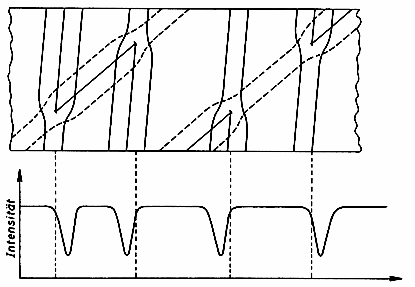
This is an important effect because it allows to analyze the nature of a dislocation loop as schematically
illustrated below. The image of the loop lies inside or outside the geometric projection; upon reversing the sign of g
or b (and this means switching from vacancy to interstitial type), the image switches between the two extremes. |
| |
|
|
 |
For a given geometry it is possible to predict if the contrast is "inside" or "outside";
the nature of a loop may thus be determined. But beware! There are many possibilities of committing a sign error! Printing
the negative with emulsion side up or down, e.g., will exchange the signs and turn a vacancy loop into an interstitial loop
or vice versa. |
 |
The next example compares regular dark field and weak-beam conditions. The object
is a very dense dislocation network with a complicated structure which we will encounter again in the context of grain boundaries. |
| |
|
|
 |
The weak-beam image on the left shows a lot more detail, but the signal to noise ratio is rather bad. This
is about the limit of the resolution obtainable under weak beam conditions. |
|
 |
In the link, a comparison between weak-beam conditions
and bright field can be found. |
 |
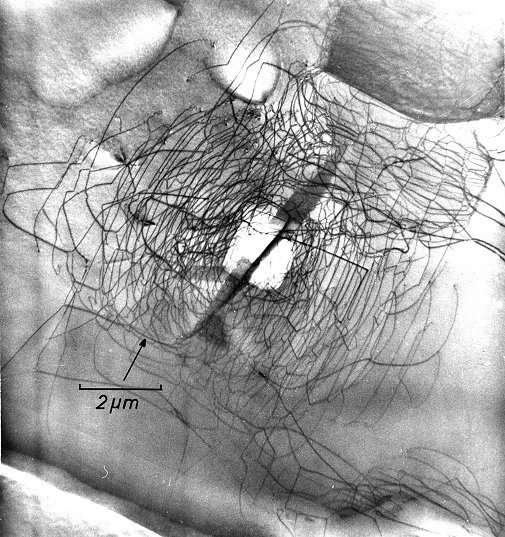
The last picture shows conventional bright field imaging. |
|
 |
The tip of a probe produced some mechanical damage in the emitter area of a transistor in an integrated
circuit (the bright square area in the center of the tangle). A microcrack was generated (the elongated black shape); upon
heating in the next processing cycle the dislocation tangle was formed to relieve the stress. |
| |
|
|
 |
By tilting the specimen while keeping the imaging conditions constant (this involves a rotation around
the diffraction vector), a second picture can be obtained under a somewhat different imaging direction. The two pictures
can be viewed in a stereo viewer and will produce the full three-dimensional glory of the structure. |
 |
More examples can be found in the links |
|
 |
Weak-beam image of a dislocation network involving partial
dislocations under different diffraction conditions
Unknown ribbon defect in Si, showing difficulties in interpretation
Radiation damage in Co showing possibilities of interpretation
FeSi precipitates in Si
Prismatic punching of dislocation loops from precipitates
Helix dislocations produced by climb of screw dislocations
Dislocations in TiAl being pinned by debris
PtSi on Si
showing the power of diffractions patterns (for another
example use the link)
Comparison weak beam - bright field |
© H. Föll (Defects - Script)