|
In a crystal
, a lattice point may be the seat of more than one atom, and the arrangement of
atoms may have a higher degree of symmetry than the lattice. |
|
 |
In Bravais lattices, which are not necessarily the primitive lattices of a crystal, this feature
expresses itself in the fact that lattice planes that do not contain lattice points
of the elementary lattice, may still contain atoms. |
|  |
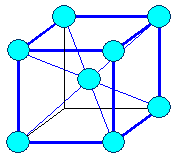
Let's illustrate this somewhat abstract concept with the familiar fcc and bcc
Bravais lattice with an atom on every lattice point |
| |
| |
| |
|
| |
Cubic primitive lattice:
Atoms are found on all
{100} planes and on every second
{200} plane. The set of {100} and {200} planes is shown on the
left and right of the drawing, respectively | |
Cubic face-centered lattice:
Atoms on all
{100} planes and on all
{200} plane with the same basic arrangement, just shifted by a/2<010> or
a/2<001>. | |
Cubic body-centered lattice:
Atoms on all {100} planes and on all
{200} plane with the same basic arrangement, just shifted by a/2<011>. |
| | |
| |
 |
In terms of defects, this feature allowed
for stacking faults in the crystals, which could
not meaningfully exist just in the lattice. |
 |
Well, the CSL lattice and the DSC lattice are lattices, after
all. But physical reality still rests with the atoms. This may have somewhat exotic consequences. |
|  |
The DSC lattice is a lattice that contains both lattices of the two crystals forming
a CSL boundary as subsets. All atoms sitting on a lattice point of the crystal
lattices therefore are also sitting on a lattice point of the DSC lattice |
|
 |
However, atoms not sitting on lattice points of the crystal
lattices, may also not sit on lattice points of the DSC lattice. In analogy to
the example above, there might be some additional symmetries hidden in the DSC lattice if we consider all atoms forming
the crystals and their positions in the DSC lattice. In particular, the stacking of planes of the DSC populated
with atoms may allow stacking faults in the DSC lattice, too, inextricably
linked to partial dislocations in the DSC lattice. |
 |
Since reality is (almost) always stranger than fiction, you should
expect that this will happen - look out for stacking faults in the DSC lattice, and, as a corollary, DSC
dislocation split into partial dislocations in the DSC lattice. |
|
 |
However, these defects in defects in defects may not be easy to find. Burgers vectors in the
DSC lattice tend to be small which makes the contrast in TEM investigations (the only method with a chance
at detecting this) rather weak. Partial DSC lattice dislocations would be even harder to see. |
|  |
Moreover, the distance between secondary dislocations in the typical networks usually encountered,
is mostly very small - there is not much room for splitting! Only in boundaries very close to a CSL orientation with
roomy networks this effect may occur. |
 |
So there is a good chance that you either never will see this or, if you see it, you may not
recognize what you see. |
|
 |
You may even, and with good justification, be of the opinion that one shouldn't even look,
because this topic is almost esoteric and is completely useless knowledge. |
|
 |
But some researchers did look and recognize - see below.
Just for the hell of it, below the head of the article is reproduced as it appeared in "Phil. Mag."; i.e the Philosophical
Magazine, which since its foundation in 1798 (which means it is one of the oldest science journals around) evolved
into one of the major scientific journals covering TEM work in general and grain boundary stuff in particular. |
| |
|
 |
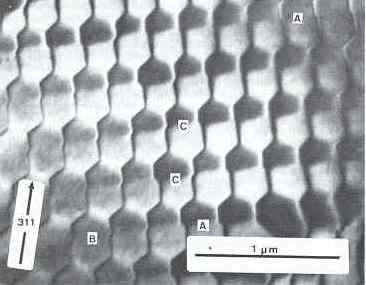
Here are two pictures of what they found. |
|
|
| |
|
|
 |
The left hand picture shows a dislocation network which is very unusual - nothing like it has
ever been observed before with regular or DSC lattice dislocations. The right hand picture shows the same network,
imaged under different diffraction conditions; the stacking faults in the DSC lattice are visible. |
© H. Föll (Defects - Script)