Figure 5.13:
Principle of osmosis.
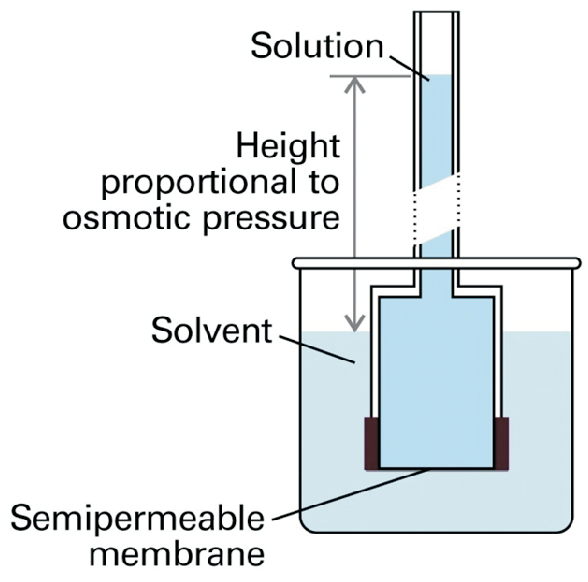
Solution and pure solute are separated at a semi-permeable membrane. Such a semi permeable membrane only allows the solvent molecules to pass. This is no problem for macromolecules, since they are large enough to fabricate a semi-permeable membrane but it becomes difficult for smaller solute molecules.
A setup to investigate osmosis is shown in Fig. 5.13. The pure solute is separated from the pure solvent by the semi-permeable membrane. If mixing is favorable the solvent will penetrate the membrane; this process will only stop when a pressure is build up to compensate for the gain in the chemical potential: Even when \(\mu(solution)\) is increasing in course of dilution, the dilution would never stop if the pressure in the solution is not increased. At equilibrium we find
|
| \begin{equation*} \mu_A^*(p)= \mu_A(solution,p+\pi) \label{eq:osmosis_eq_1} \end{equation*} | (5.46) |
Typical approximations for easy quantification of osmotic pressure are
No exchange of solute is assumed.
The dilution of the solution (close to membrane) by the solvent is neglected for the quantification.
Osmosis is very significant for the determination of molar masses of polymers and proteins
which have low density and show no dissociation.
As an example for the impact of osmosis one can take
Mg implants: they are rapidly resolved, thus, the concentration of Mg salt in the surrounding is critically high. In consequence
an osmotic death of cells is observed.
 |
 |
 |
 |
© J. Carstensen (TD Kin I)