Potential Discontinuities and Dipole Layers

The Poisson equation in its simplest form reads

e₀e _r ·	d²V dx²	= –	r(x)

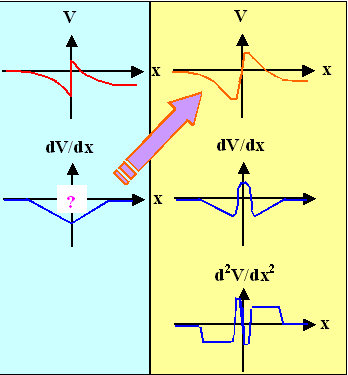
Differentiating the potential V including possible discontinuities thus will gives us the charge distribution r( x). We can do that very easily in a qualitative way as shown on the left hand side below.

Note that an infinitely sharp discontinuity will not be noticed in the dV/dx curves. The curves we get are identical to the old curves that did not contain a discontinuity.

But infinitely sharp discontinuities, or singularities in general, mostly do not make sense in physics. All we have to do therefore, is to redraw the potential with the discontinuity spread over a small distance (obviously in the order of the atom size at the very minimum)

Differentiating graphically in a qualitative way now is easy, this is shown on the right hand side.

We now get a sharp "wiggle" in the charge distribution, corresponding to a dipole layer of charge right at the interface.

	Much can be learned from this. Here are a few suggestions for investigations of your own:
		Look at the other type of discontinuity.
		Look at the case of extremely heavily doped semiconductors
		Now look at the junction between two different metals. Can you understand why such a junction is not "felt" electronically?
		Can you guess on how much charge is transferred from one material to the other one? On the field strength that we encounter in these dipole layers?

With frame

5.3.1 Ideal Heterojunctions

Basic Equations

Solving the Poisson Equation for p-n-Junctions

5.3.3 Real Heterojunctions