Examples: Scalar product

2.14.1 Examples: Scalar product

Let

\[\begin{array}{cc} \vec{a}= \vect{a_1\\\vdots\\a_n}\;\in\mathbb{R} & \qquad \vec{b}=\vect{b_1\\\vdots\\b_n}\;\in\mathbb{R} \end{array}\]

be two vectors with real components:

Definition 29 $\vec{a}\cdot\vec{b}=a_1b_1+\ldots+a_Nb_N=\sum_{j=1}^k a_j b_j \;$ is called scalar product of $\vec{a}$ and $\vec{b}$
Note: $\vec{a},\vec{b}\;\in\mathbb{R}^N, \vec{a}\cdot\vec{b}\;\in\mathbb{R}$!!

Example:

\[\begin{array}{lccccccccl} \vec{a}&=&\vect{1\\2\\3\\4},&\vec{b}&=&\vect{5\\6\\7\\8}&\Rightarrow& \vec{a}\cdot\vec{b}&=&5+12+21+32\\ &&&&&&&&=&70\\ \vec{a}&=&\vect{1\\-1\\1},&\vec{b}&=&\vect{1\\1\\0}&\Rightarrow&\vec{a}\cdot\vec{b}&=&1-1+0=0\\ \end{array}\]

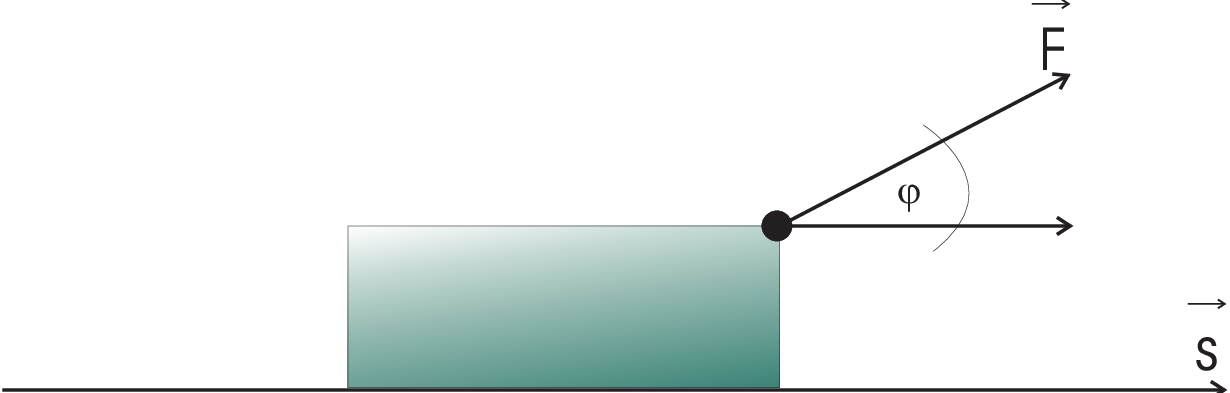
Example from physics:
Work:

\[W = \vec{F}\cdot\vec{s} = \left|\vec{F}\right|\;\left|\vec{s}\right|\cdot\cos\varphi\]

\[\left.\begin{array}{lcl}\cos\angle\left(\vec{a},\vec{b}\right)&=&\mbox{\large $\frac{\vec{a}\cdot\vec{b}}{\left|\vec{a}\right|\cdot\left|\vec{b}\right|}$}\\\\ \vec{a}\cdot\vec{b}&=&0\quad\text{if}\quad \vec{a} =\vec{0} \quad\text{or}\quad \vec{b}=\vec{0} \quad\text{or}\quad \vec{a}\bot\vec{b}\end{array}\right\}\,\mbox{in 3D}\]

now definitions for $\vec{a},\vec{b}\,\in\mathbb{R}^N$

$\vec{a},\vec{b}\neq\vec{0}$. If $\vec{a}\cdot\vec{b}=0$ then $\vec{a}$ is called orthogonal or perpendicular to $\vec{b}$ or $\vec{a}\bot\vec{b}$
$\frac{\vec{a}\cdot\vec{b}}{|\vec{a}|\,|\vec{b}|}\le1$. We define $\cos\varphi=\frac{\vec{a}\cdot\vec{b}}{|\vec{a}|\,|\vec{b}|}$ where $\varphi$ is the angle between the two vectors $\vec{a}$ and $\vec{b}$ in $\mathbb{R}$

Example:

\[\vec{a} = \vect{1\\1\\1} \quad \vec{b} = \vect{2\\2\\0}\quad\Rightarrow\quad\cos\varphi=\frac{2+2+0}{\sqrt{3}\sqrt{8}}=\sqrt{\frac{2}{3}}\rightarrow\,\varphi=0.615=35.26^\circ\]
\begin{eqnarray*}\vec{a}&=&\begin{array}{crcc}\vect{1\\1\\1\\1}&\vec{b}&=&\vect{2\\2\\0\\0}\end{array}\Rightarrow\,\cos\varphi=\frac{2+2+0+0}{\sqrt{4}\sqrt{8}}=\frac{1}{\sqrt{2}}\,\Rightarrow\,\varphi=\frac{\pi}{4}=45^\circ\\ \vec{a}&=&\begin{array}{crcc}\vect{1\\1\\1\\1}&\vec{b}&=&\vect{1\\-1\\-1\\1}\end{array}\Rightarrow\,\vec{a}\cdot\vec{b}=1-1-1+1=0\,\rightarrow\,\vec{a}\bot\vec{b} \end{eqnarray*}

With frame

Scalar product