|
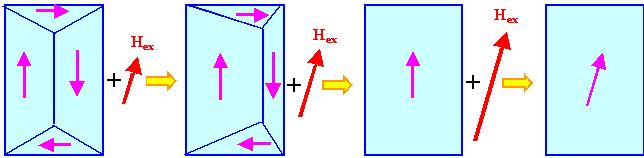
What happens if we apply an external field to a ferromagnet with its equilibrium
domain structure? |
|
 |
The domains oriented most closely in the direction of the external field will
gain in energy, the other ones loose; always following the basic equation for
the energy of a dipole in a field. |
|
 |
Minimizing the total energy of the system thus calls for increasing the size of
favorably oriented domains and decreasing the size of unfavorably oriented ones. Stray field considerations still apply,
but now we have an external field anyway and the stray field energy looses in importance. |
|
 |
We must expect that the most favorably oriented domain will win for large external
fields and all other domains will disappear. |
|
 |
If we increase the external field beyond the point where we are left with only
one domain, it may now even become favorable, to orient the atomic dipoles off their "easy" crystal direction
and into the field. |
|
 |
After that has happened, all atomic dipoles are in field direction - more we cannot
do. The magnetization than reaches a saturation value that cannot be increased anymore. |
 |
Schematically, this looks like as shown below: |
| |
|
|
 |
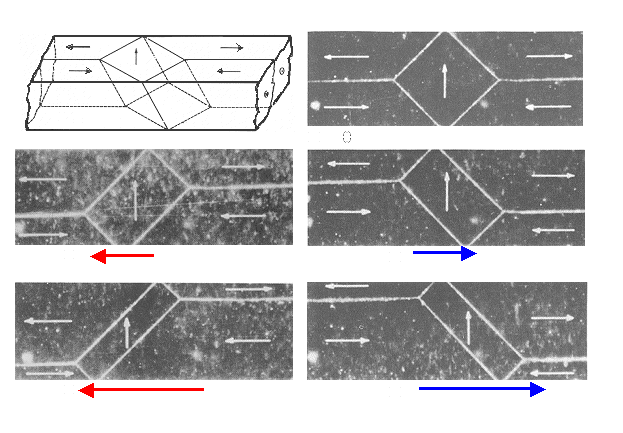
Obviously, domain walls have to move to allow the new domain structure in an external
magnetic field. |
 |
What this looks like in reality is shown below for a small single crystal of iron. |
| |
|
|
 |
As noted before, domain walls interact with stress
and strain in the lattice, i.e. with defects of all kinds.
They will become "stuck" (the proper expression for things like that is "pinned")
to defects, and it needs some force to pry them off and move them on. This force comes from
the external magnetic field. |
 |
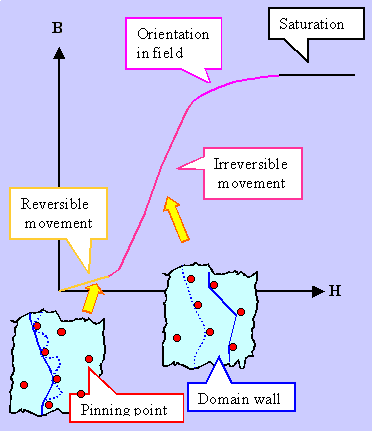
The magnetization curve that goes with this looks like this: |
| |
|
| |
|
 |
For small external fields, the domain walls, being pinned at some defects, just bulge out
in the proper directions to increase favorably oriented domains and decrease the others. The magnetization (or the magnetic
flux B) increases about linearly with H |
 |
At larger external fields, the domain walls overcome the pinning and move in the right direction
where they will become pinned by other defects. Turning the field of will not drive the walls back; the movement is irreversible. |
 |
After just one domain is left over (or one big one and some little ones), increasing the field
even more will turn the atomic dipoles in field direction. Since even under most unfavorable condition they were at most
45o off the external direction, the increase in magnetization is at most 1/cos(45o) = 1.41. |
 |
Finally, saturation is reached. All magnetic dipoles are fully oriented in field direction,
no further increase is possible. |
|
| |
 |
If we switch off the external field anywhere in the irreversible region, the domain
walls might relax back a little, but for achieving a magnetization of zero again, we must use force to move them back, i.e.
an external magnetic field pointing in the opposite direction. |
|
 |
In total we obtain the well known hysteresis
behavior as shown in the hysteresis curve below. |
| |
|
| |
|
 |
The resulting hysteresis curve has two particular prominent features:
- The remaining magnetization for zero external field, called the remanence
MR, and
- the magnitude of the external field needed to bring the magnetization down to zero again. This is called coercivity
or coercive field strength HC.
|
 |
Remanence and coercivity
are two numbers that describe the major properties of ferromagnets (and, of course, ferrimagnets, too). Because the exact
shape of the hysteresis curve does not vary too much. |
 |
Finally, we may also address the saturation magnetization
MS as a third property that is to some extent independent of the other two. |
| |
|
|
 |
Technical optimization of (ferro)magnetic materials first always focuses on these
two numbers (plus, for reasons to become clear very soon, the resistivity). |
|
 |
We now may also wonder about the dynamic behaviour, i.e. what happens if we change
the external field with ever increasing frequency. |
|
| |
|
Domain Wall Structure |
| |
|
 |
The properties of the domain walls, especially their interaction with defects
(but also other domain walls) determine most of the magnetic properties of ferromagnets. |
|
 |
What is the structure of a domain wall? How can the magnetization change from
one direction to another one? |
|
 |
There are two obvious geometric ways of achieving that goal - and that is also what really
happens in practically all materials. This is shown below. |
| |
|
 |
What kind of wall will be found in real magnetic materials? The answer, like always
is: Whichever one has the smallest (free) energy |
|
 |
In most bulk materials, we find the Bloch wall: the magnetization
vector turns bit by bit like a screw out of the plane containing the magnetization to one side of the Bloch wall. |
|
 |
In thin layers (oft the same material), however, Neél walls
will dominate. The reason is that Bloch walls would produce stray fields, while Neél walls can contain the magnetic
flux in the material. |
 |
Both basic types of domain walls come in many sub-types, e.g. if the magnetization
changes by some defined angle other than 180o. In thin layers of some magnetic material, special domain
structures may be observed, too. |
 |
The interaction of domain walls with magnetic fields, defects in the crystal (or
structural properties in amorphous magnetic materials), or intentionally produced structures (like "scratches",
localized depositions of other materials, etc., can become fantastically complicated. |
|
 |
Since it is the domain structure together with the response of domain walls to these interactions
that controls the hystereses curve and therefore the basic magnetic properties of the material, things are even more complicated
as described before. |
|
 |
But do keep in mind: The underlying basic principles is the minimization of the free enthalpy,
and there is nothing complicated about this. The fact that we can no easily write down the relevant equations, no to mention
solving them, does not mean that we cannot understand what is going on. And the material has no problem in solving equations,
it just assumes the proper structure, proving that there are solutions to the problem. |
| |
|
© H. Föll (Electronic Materials - Script)