|
High-Resolution TEM (HRTEM) is the ultimate tool in imaging defects. In favorable
cases it shows directly a two-dimensional projection of the crystal with defects and all. |
|
 |
Of course, this only makes sense if the two-dimensional projection is down some low-index
direction, so atoms are exactly on top of each other. |
 |
The basic principle of HRTEM is easy to grasp: |
|
 |
Consider a very thin slice of crystal that has been tilted so that a low-index direction is exactly perpendicular
to the electron beam. All lattice planes about parallel to the electron beam will be close enough to the Bragg position
and will diffract the primary beam. |
|
 |
The diffraction pattern is the Fourier
transform of the periodic potential for the electrons in two dimensions. In the objective
lens all diffracted beams and the primary beam are brought together again; their interference provides a back-transformation
and leads to an enlarged picture of the periodic potential. |
|
 |
This picture is magnified by the following electron-optical system and finally seen on the screen at magnifications
of typically 106. |
 |
The practice of HRTEM, however, is more difficult them the simple theory.
A first illustration serves to make a few points: |
|
|
|
|
 |
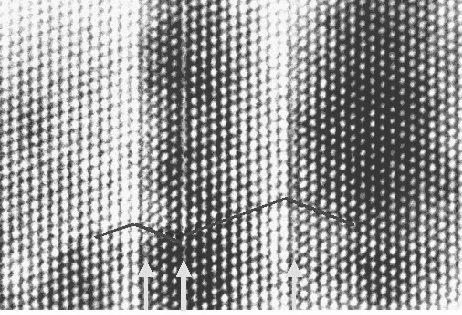
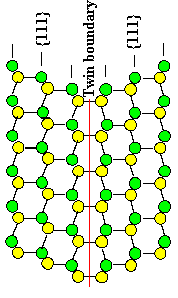
The image shows one of the first HRTEM images taken around 1979; it is the <110>
projection of the Si-lattice; a schematic drawing is provided for comparison. It also contains a few special grain
boundaries, called twin boundaries. |
 |
We notice a few obvious features: |
|
 |
Instead of two atoms we only see a dark "blob." |
|
 |
Or does the dark blob signal the open channels in the lattice projection? There is actually no way of telling
from just one picture. |
|
 |
The twin boundaries look fine in comparison to the drawing at a first glance. Looking more closely, one
realizes that there are a few unclear points: The yellow arrow points to "fuzzy" lattice planes to the right (or
left) of the boundary. Following a fringe across the boundary seems to result in an offset - what does it mean? But what
should we expect defects (in this case the twin boundaries) to look like? After all, they destroy the periodicity of the
lattice and it is not obvious what Fourier transforms of defects will produce in general cases. |
 |
The last point is easy to solve: Just do a simulation of a defect (i.e. calculate
the image for an assumed slice of a crystal with all atoms at the proper positions), but mind the points mentioned below!
These are the limitations to HRTEM stemming from the non-ideality of the instrument and the specimen: |
|
 |
The specimen is not arbitrarily thin! If the thickness is in the order of the extinction length, some reflexes
may have very small intensities because they were diffracted back into the primary beam. The objective lens then will not
be able to reconstruct the spatial frequencies contained in these reflections; the image looks like a different lattice. |
|
 |
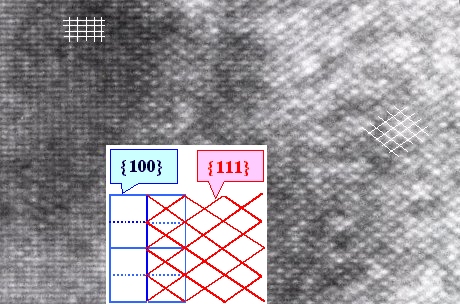
This can be nicely seen in a HRTEM image of Si where the thickness of the sample increases
continuously: |
| |
|
|
 |
The inset shows the lattice in <110> projection; an elementary cell is given by the large
rectangles formed by solid blue lines. |
|
 |
On the right hand side of the picture, all reflections are excited; the very strong {111} reflections
dominate the image and the {111} lattice planes (indicated by white lines) are most prominent. On the left hand side
the thickness happens to be in a region where the {111} reflections are weak; the {400} type reflections dominate
({100} etc. are "forbidden" in the diamond lattice). The lattice appears rectangular. |
 |
In principle, this can be calculated, too, without much problems. What is much
more problematic is the "contrast transfer function" of the objective lens.
|
|
 |
If we consider the objective lens to be some kind of amplifier that is supposed to amplifies (spatial)
frequencies in the input with constant amplification and without phase distortion, the objective lens is a very
bad amplifier. It has a frequency response that is highly nonlinear, the amplification drops off sharply for
high (spatial) frequencies (meaning short distances). In other word, the resolution is limited (to roughly 0,1 nm
in good TEMs); you cannot see smaller details. |
|
 |
But worse yet, around the resolution limit, the objective lens induces strong phase shifts as a function
of several parameters (the most important one being the focus setting); this influences the interference pattern which will
define the image. |
 |
Both effects together can be expressed numerically in the contrast transfer function
of the lens. If you know that function (for every picture you take) you may than calculate what the image would look like
for a "perfect" lens with a certain resolution limit; or somewhat easier, you calculate what a crystal with the
defects you assume to be present would look like in your particular microscope with the contrast transfer function that
it has. |
 |
Neither approach is very easy; the amount of computing needed can be rather large.
Worse, you must determine parts of the contrast transfer function experimentally; and that involves taking several images
at different focus settings. Still, HRTEM images provide the ultimate tool for defect studies. They are perfectly
safe to use without calculations if you obey two simple rules: |
|
 |
Only look at pictures where the perfect part of the crystal looks as it should. After all, you usually
know what kind of material you are investigating. So if the image of a diamond structure looks like the left part of the
illustration above; throw it away (or at least use with care). It it looks like a diamond strucure you can't go totally
wrong in interpreting the picture. |
|
 |
Only draw qualitative conclusions (e.g. there is a dislocation in this GaAs specimen!); never draw
quantitative conclusion (e.g. it ends at a Ga atom!) without calculating the image. |
 |
Some more details to HRTEM imaging can be found in the (German) article in the link |
 |
Three examples may serve to illustrate HRTEM here; more will be found in
the upcoming chapters. |
|
 |
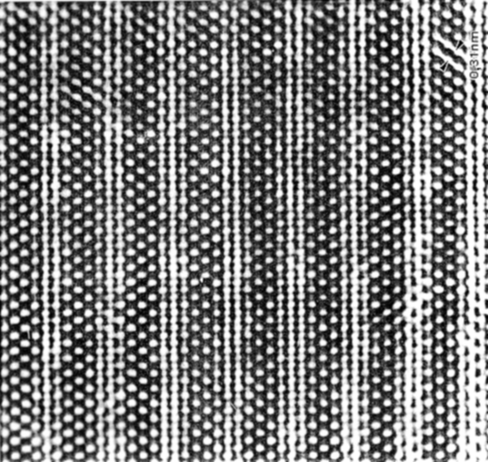
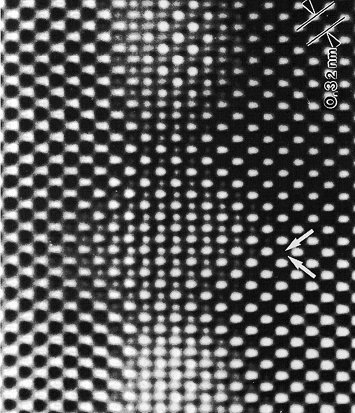
The first picture shows a small angle grain boundary in Si. This was the first picture
of this kind; it only can be interpreted qualitatively; the contrast transfer function was not known. What we see beyond
doubt are several lined-up dislocations which constitute a boundary - the top half of the lattice is tilted with respect to the bottom half.
|
| |
|
|
 |
The next picture (from W. Bergholz) shows an SiO2 precipitate in Si. Again, a
qualitative interpretation is neither possible nor necessary. It is clear that the precipitate, albeit very small, is not
spherical |
| |
 |
| Courtesy of W. Bergholz |
|
|
 |
The last example shows quantitative HRTEM (from W. Jäger)
Careful imaging under various conditions, extraction of the contrast transfer function and prodigious computing allowed
not only to image a sequence of Si - Ge multilayers produced by molecular beam epitaxy (MBE), but to identify the
positions of the Si and Ge atoms. The first picture shows an overview. The brighter regions indicate the Ge
layers, but it is not clear exactly how the lattice changes from Si to Ge. |
| |
 |
| Courtesy of W. Jäger |
|
| | |
|
 |
This image also demonstrates the progress made in building electron microscopes. The "old" pictures
shown above were taken with a the best general-purpose TEM available around 1980 (Siemens Elmiskop 102).
The last pictures were taken with a TEM optimized for high resolution around 1995. |
 |
Next a comparison between an enlarged part of the Ge/Si stack is shown together with
a quantitative evaluation of this and other pictures obtained at different focus settings from W. Jaeger and his group.
The color codes defined Ge concentrations and a very clear representation of the multilayer sequence is obtained. |
| |
 |
 |
| Courtesy of W. Jäger |
|
© H. Föll (Defects - Script)