5.2.4 Forces on Dislocations

Again, detailed calculations are complicated and must be done numerically in most cases. For practical usage, however, we will find simple approximations by using the energy formula already derived; this will be good enough for most cases.

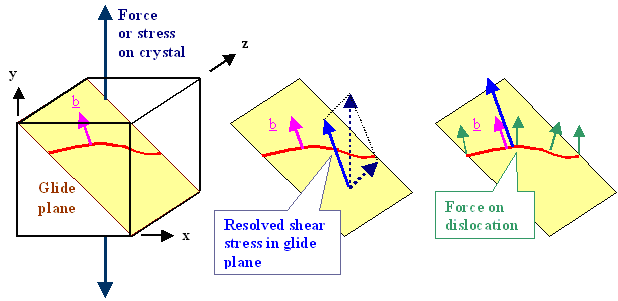
First, we have to see that for the movement of a dislocation on its glide plane, we only need to consider the shear stress on this plane. This is so, because only force components lying in the glide plane of the dislocation can have any effect on dislocation motion in the glide plan. The normal components of the the stress in the glide plane system act perpendicular to the glide plane and thus will not contribute to the dislocation movement.

Both shear stress components in the glide plane act on the dislocation. Important, however, is only their combined effect in the direction of the Burgers vector, which is called the resolved shear stress t_res; for simplicity it will just be called t from now on.

However, while the resolved shear stress points into the direction of the Burgers vector, the direction of the force component acting on, i.e. moving the dislocation, is always perpendicular to the line direction! This is so because the force component in the line direction does not do anything - a dislocation cannot move in its own direction. or, if you like that better: If it would - nothing happens! The whole situation is outlined below

Under the influence of the force F acting on the dislocation and which we want to calculate, the dislocation moves and work W is done given by W = Force · distance. Lets look at the ultimate work that can be done by moving one dislocation.

If the dislocation moves in total through the crystal on a glide plane with the area A, the upper half of the crystal moves by b relative to the lower half which is the distance on which work has been done.

This only happens if a shear force acts on the crystal, and this force obviously does some work W. This work is done bit by bit by moving the dislocation through the crystal, so we must identify the force that does work with the force F acting on the dislocation.

The acting shear stress in this case is then t = Force F/area A. and force F is that component of the external force that is contained or "resolved" in the glide plane of the dislocation as discussed above.

For the total work W done by moving half of the crystal a distance equal to the Burgers vector b we obtain

W	=	A · t · b

With A · t = Force; b = Burgesvector = distance.

We just as well can divide W into incremental steps dW, the incremental work done on an incremental area that consists of an incremental piece dl of the dislocation moving an incremental distance ds, as shown below

The relation between the incremental work dW to the total work W then is just the ratio between the incremental area to the total area; we have

dW W	=	ds · dl A

Putting everything together, we obtain

dW	=	A · t · b ·	dl · ds A	=	t · b · dl · ds

An incremental piece of work dW can always be expressed as a force times an incremental distance ds; i.e. dW = F · ds. The force F acting on the incremental length dl of dislocation then obviously is F = t · b · dl.

If we now redefine the force on a dislocation slightly and refer it to the (incremental) unit length dl, i.e. we take F* = F/dl, we obtain a very simple formula for the magnitude of the force (it is not a vector!) acting on a unit length of a dislocation:

F* = t · b

This is easy - but beware of the sign of the force! You must get all the signs right (Burgers vector, line vector, t) to get the correct sign of the force! We also will drop the "*" in what follows, because as with all other properties of dislocations, it is automatically per unit length if not otherwise specified.

The important part is t. It is the component of the shear strain in the glide plane in the direction of b. This is normally not a known quantity but must be calculated, e.g. by a coordinate transformation of a given external stress tensor to a coordinate system that contains the glide plane as one of its coordinate planes.

Again, we must realize that the Force F as defined above is always perpendicular to the dislocation line; even if t = constant everywhere on the glide plane.

This is somewhat counterintuitive, but always imagine the limiting case of a pure edge and screw dislocation: The same external t must exert a force on a screw dislocation that is perpendicular to the force on an edge dislocation to achieve the same deformation (think about that; looking at the pictures helps!)

This calls for a little exercise

Exercise 5.2-2
Forces on a dislocation

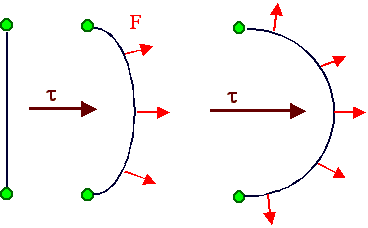
In reality, dislocations can rarely move in total because they are usually firmly anchored somewhere. For a straight edge dislocation anchored at two points (e.g. at immobile dislocation knots) responding to a constant t, we have the following situation.

The forces resulting from the resolved shear stress (red arrows) will "draw out" the dislocation into a strongly curved dislocation (on the right). A mechanical equilibrium will be established as soon as the force pulling back the dislocation (its own line tension) exactly cancels the external force.

The middle picture shows an intermediate stage where the dislocation is still moving.

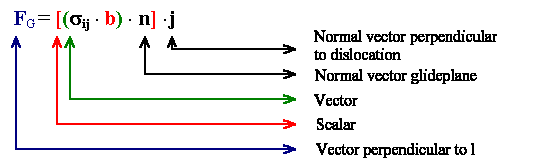
It is possible to write down the force on a dislocation as a tensor equation which automatically takes care of the components - but this gets complicated:

First we need to express the force as a vector with components in the glide plane and perpendicular to it. We define F = Force on a dislocation = (F_N, F_G)

With F_G = component in the glide plane, F_N = component vertical to the glide plane. Only F_G is of interest, it is given by

Note that scalars, vectors and tensors are combined to form ultimately a vector. The colors of the brackets code the respective property as outlined in the margin.

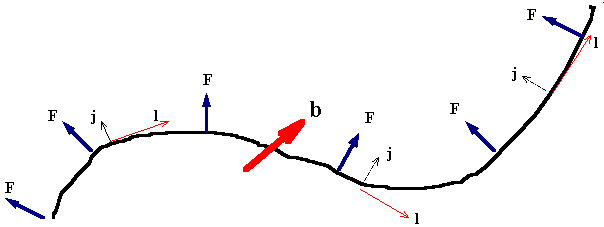
The consequences of this equation and the quantities used are illustrated below. Also note that you have many ways to confuse signs!

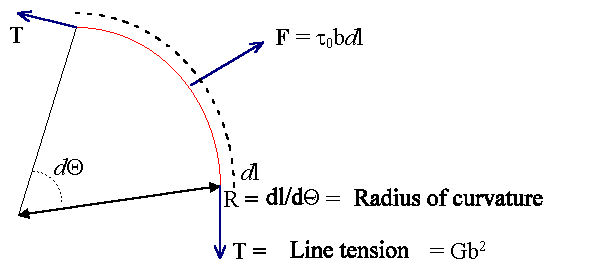
Using the formulas derived so far, we can find an important quantity, the shear stress necessary to maintain a certain radius of curvature for a dislocation.

If we look at the illustration above, we see that for a certain stress, the force will draw the dislocation into a curved line, but for some configuration there will be a balance of force, because the line tension of the dislocation pulls back.

We can calculate the balance of power by looking at an incremental piece of dislocation with a radius of curvature R. The acting force F is balanced by the line tension T.

Let's assume we increase the radius R of an incremental curved piece by dl. The acting force needed for this is F = t · b · dl and we have dl = R · dQ. The picture below shows a very large dQ for clarity.

The line tension T = Gb² is "pulling back", but only a small component T_–F is directly opposing F.

The component T_–F is given by

T	= T · sin(dQ/2) » dQ/2		for small dQ

Since there are two components we have the balance of power

T · dQ = Gb²· dQ = t · b · dl = t · b ·R · dQ

The equilibrium radius R₀ obtained for a shear stress t₀ is thus

R₀ =	Gb t₀

This will have important consequences becuase the equation states that a dislocation will move "forever" if t > Gb/R_min with R_min denoting some minimal radius of curvature that cannot be decreased anymore.

From looking at force balance, we now can answer the questions posed before for a dislocation network:

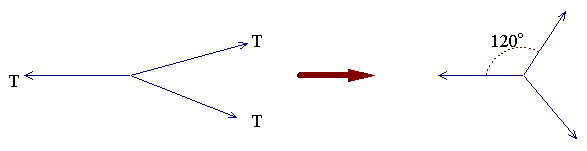
The sum of the line tensions at a knot must be zero, too (or at least very small), otherwise the knot and the dislocations with it will move. We thus expect that 3-knots will always show angles of (approximately) 120^o.

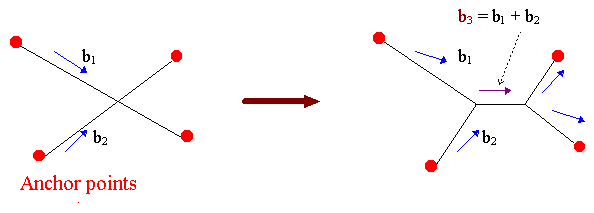
Knots with more than three dislocations will, as a rule, split into 3-knots, since otherwise there can be no easy balance of line tensions. In real cases, however, you must also consider the geometry of the anchor points (are they fixed, can they move?), the change of line energy with the character of the dislocation and the new total length of the dislocations.