Nucleation
and Propagation of Misfit Dislocations in Strained Epitaxial Layer Systems
P.B. Hirsch
(Sir Peter)
Department of Materials, University of Oxford
The annotated version
Comments on light blue background |
1. Introduction
In 1949
Frank and van der Merwe [1] discussed theoretically the stresses and the energies at the interface
of an epitaxial layer grown on a matrix with a slightly different lattice parameter. Their
one-dimensional model was extended to two dimensions by Jesser et al.[2]. These studies show
that if the lattice mismatch is small, and/or the thickness of the overlayer is not large,
the growth of the epilayer is pseudomorphic (commensurate) with the matrix, with the atomic
planes on the two sides of the interface being in perfect register with each other. The mismatch
is accommodated by an elastic strain in the epilayer giving a biaxial stress
of [3]
| s | =
| 2m f · (1 + n)
(1 – n
) | | (1) |
where m is the shear modulus, n Poisson's
ratio and f the misfit parameter. Elastic isotropy is assumed. The misfit parameter
is given by f=(ae -am)/am, where ae
, am are the lattice parameters of the unconstrained epilayer and matrix
in the plane parallel to the interface.
 |
How did he get this formula? Well, this is easy, but deriving the starting formula also illustrates
a certain problem one might encounter from looking at simple pictures all the time. Let's see: |
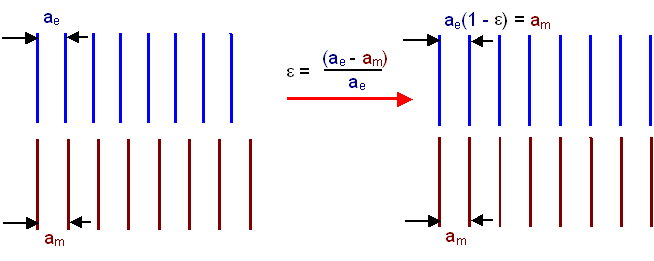
|  | If you strain the lattice of the epitaxial layer
in one dimension, so that it fits the matrix perfectly, you have the following situation: |
| |
| | | |
|
| |
| |  | The strain eneeded for perfect fit is e=(ae – am)/ae; but since
ae and am are nearly equal, dividing by ae
(as is correct) or by am (as Sir Peter does) makes no difference. So we can
equate e with f=(ae – am)/am
, the misfit parameter according to Sir Peter. |
| |
 |
Strain e and stress s
are usually related by
s=E· e
with E=modulus of elasticity (Youngs modulus).
But since E
can be expressed in terms of the shear modulus mand Poissons
ratio n by
E=2m(1 + n)
, we can write
s=2m(1 + n) · e=2m(1
+ n) · f
|
|  | Apart from the factor (1 – n),
this is Sir Peters starting formula. Where does the (1 –
n) come from? |
 |
Let's look at the problem carefully. We actually have a two-dimensional
problem and must consider biaxial
stress. Our simple figure was one-dimensional - and that is where we might have missed
something. |
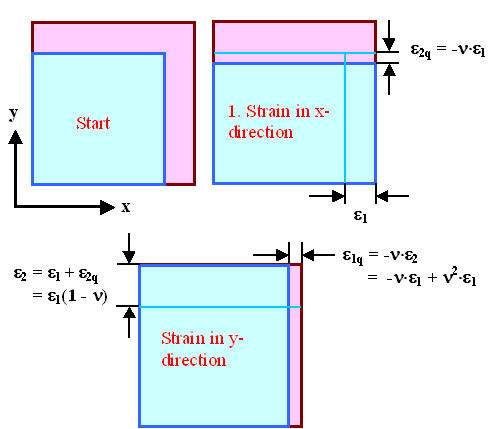
|  | Did we miss something? Well,
yes - we did. In the picture above, we have applied a suitable stress to strain the blue lattice
to the desired value in the x-direction. If we now apply the same stress in the
y-direction perpendicular to the first one, we will have to use a larger strain
because the y-dimension of the crystal layer will now be smaller as expressed
by Poissons modulus. This is illustrated below. |
| | |
| |
|
|
| |
|
 | After
we pulled the blue crystal sheet to the desired dimension by a strain e
1, its lateral dimension in y direction decreased by e2qas shown. We now must apply a strain of
e2=e1(1 – n)
to make the match in y-direction. |
|  | That's
it. For reasons of symmetry, this must be the strain corrected for
biaxial stress in both directions, i.e. we have
e1
=e1,2/(1 – n)
This is the expression used by Sir Peter. |
|  | Of
course, we made a little mistake. The deformation in y-direction will lead to
a shrinkage in x -direction which me must compensate, which in turn will lead
to a shrinkage in y-direction, which will lead to a shrinkage - and so on ad
infinitum. |
|  | But all this does is to add
higher order terms in n which we commonly neglect in linear
elasticity theory. |
For the case of GexSi1-x
alloys Vegard's law is approximately obeyed [4], i.e. aGeSi=aSi+(aGe
- aSi)x, where the a's are the lattice parameters. This means that for GexSi
1-x epilayers on an Si(001) surface, f is a linear function of x; since the
lattice parameter of Ge (0.5657nm) is greater than that of Si (0.5431nm), f increases
with x (f(x)=0.042x), and the epilayer is in compression. Beyond a critical strain and/or
thickness it becomes energetically favourable for the misfit to be accommodated by a network
of interface dislocations. In view of the importance of strained epilayers or superlattices
for device applications [5] and the development of methods of growing them, much research.
has been devoted in recent years to the conditions which control the relaxation of elastic
strain by the introduction of misfit dislocations, and to the mechanisms by which they are
formed. This paper presents a brief review of this field of research; it does not pretend
to be exhaustive.
2. Energetic Considerations
The energy of the system involving
a strained epilayer and an array of misfit dislocations is generally discussed for the case
of the layer and matrix material being cubic, and the interface parallel to a cube plane.
The energy per unit area of a square grid of edge dislocations, Burgers vector b, with
dislocation spacing p is given approximately by
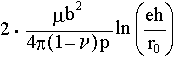 | (2') |
where h is the film
thickness, r0the core radius, and where the factor 2 arises because
of the presence of two orthogonal sets of edge dislocations.

| First, there is a slight correction
in eq: (2): The "x" after the "2" as shown in the original has been replaced by a dot - because the
"×", writtenas "x" as a sign for multiplication is no
longer allowed; it has been used up as denoting the amount of Ge in the alloy Gex
Si1 – x . |
 | Now the formula
contains an unexplained "e". Since we know that the energy of a dislocation
contains the term ln(R/r0) with R being some outer radius, it is clear
that R cannot be larger than h, the thickness of the layer. But by simply equating
R with h, we make some numerical mistake which we might correct by introducing
a unspecified (but probably not very large) correction factor
e? |
|  | That was my first thought.
Well - wrong! Sir Peter simply takes one of the many formulas
for the total energy of a dislocation that float around, it is the same formula as shown before (for the purpose of recalling it here), and e
is e indeed - the base for natural logarithms. |

| We then have the correct formula except
for the 2/p. But this is easy and pointed out (albeit somewhat obliquely) by Sir
Peter: |
| |  | The general formula for the
dislocation energy gives the energy per unit length of
the dislocation. If we want the energy per unit area, we
have to multiply by the length of the dislocations in an unit areas and then divide by the
unit area. |
| |  | If we take the unit area to
be p2, the areas of one cell of the (square) dislocation network, it contains
dislocations with the length 2p- we have the factor 2/p. |
The elastic strain remaining is given by e=f – b/p.
 | This is something easy to figure out for yourself. Just
take into account that every dislocation with a Burgers vector b relaxes the
total deformation by one b(provided it is fully contained in the plane of the boundary). |
|  | Full relaxation thus would occur if a misfit dislocation
network with a spacing p=b/f=b/(ae – am)/am is
introduced which partially relaxes the epitaxial layer. |
|
 |
The essential trick is to generate a variable
e which is the residual strain
contained in a partially relaxed epitaxial layer. So some
of the strain and its energy is gone, but at a cost: Dislocations, carrying their own energy
penalty, are introduced. |
|  | Since e is a variable, it can now be used to optimize the system as we will
see. |
The total energy per unit area E is then given by
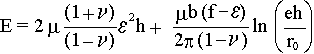 | (3) |
where the
first term is the elastic strain energy.
 |
Here Sir Peters gets a bit tricky once more. The elastic energy Eelast of
an uniaxially elastically deformed material is simple s·e/2. For biaxial strain its twice that, and that gives the first term. |

| The second term is the energy of the dislocation network,
it is almost the formula from above. |
|  | However, p has been replaced
using the relation
e=f – b/p, or
p=b/
(f – e). |
 | The
next part is straight forward. The total energy per unit area is a function of
e, the strain still present in the epitaxial layer even after some dislocations
have been introduced. |
|  | So we can find
the minimum energy of the system with respect to e by calculating
dE/de =0. |
|  | The calculation is straight forward: |
For a given thickness
the minimum energy occurs for a value e0 given
by
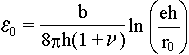 | (4) |
If e0 = f,
then the layer is ideally commensurate with the substrate, and
the elastic strain is equal to f. If e0 < f
then some misfit will be relaxed by dislocations, the spacing being given by (5) with e0 =f – b/p. The critical film thickness, hc
, at which it becomes energetically favourable for the first dislocation to be introduced
is obtained with e0=f, i.e.

| Got it? Well, lets look at the argumentation in detail? |
|  | If the remaining
strain e0 =f, then it is the strain
of the unrelaxed layer and the formula defining e yields b/p=0 which, since b has a defined value, can
only mean p is infinite - in other words there is no dislocation network. |
|  | If e0 > f, the layer
is not ideally commensurate with the substrate as stated
in the original, but a dislocation
network must be present (b/p < 0 is required) which increases the strain
- the sign of b is the wrong way around. This is of course a totally unphysical
high energy situation, and we can safely exclude e0
> f from the possible range of e values. |
|  | If e0 < f, again
a dislocation network must be present, but this time with the right sign of b
- it decreases the total elastic strain. |
 | Now, e0 , the optimal residual strain is a function of the layer
thickness h. It decreases with increasing h and this means that dislocations
must be introduced at some critical thickness hc. |
|
| 
| If the thickness is below hc, we have no dislocations
and e=f obtains. |
|  | If the thickness is above hc, we have dislocations and e < f obtains. |
 | This
leaves us with e0 =f at the point of critical
thickness. All we have to do now is to express the equation above for h; it will then
give hc by substituting f for e0
. |
|  | Sir Peter now writes |
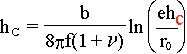 |
(5) |
 | Two points should be made about this relation: |
| |
 | First, the above version of equation (5) corrects the
little mistake of the original of forgetting
the index "c" at the h in the argument of the logarithm, and |
| |  | Second;
this equation is now a transcendent equation for hc
; we cannot write it down in closed form. |
Two points should be
made about this relation. First, hc depends on the core radius r0
(» b), and the uncertain value of this parameter introduces
some uncertainty into this relation, particularly for small h/r0. Secondly,
hc depends on the assumed dislocation arrangement; for example the misfit
might be relieved by dislocations with different b; eqn. (2) shows that the dislocation strain
field energy is smaller for edge dislocations of smaller energy, even though for the same
relief of strain (f – e), the spacing p will
be smaller. Thus it is necessary to take care in making comparisons between theory and experiment.
In practice, however, it is generally found that the observed values of hc
are larger than those predicted over most of the range of misfits (see for example People
and Bean [6] for Ge-Si layers on (100) Si). The reasons for this discrepancy
are partly due to insensitivity of the experimental techniques used, and partly kinetic in
origin. In order to introduce dislocations, there have to be mechanisms for doing so, and
for most practical cases, except these with very large misfits, the strain relief is limited
by kinetic considerations.
 | Now, what order of magnitude do we get for hc, forgetting about
the small detail of unclear core radii and so on? |
|
 |
Well, if we rewrite the above equation, with 8p(1
+ n) » 30, and ln ehc/r
0=y, we have
hc » b/f · (ln
y)/30 |
|  | How large is ln y?
If we take r0 to be about 0,3 nm, and ehc to be
anywhere between 10 nm and 1000 nm, we have a range of values from ln(10/0,3)=3,
51 to ln(1000/0,3)=8,11). |
|  | In
other words, it doesn't matter much for orders of magnitude. Lets take an intermediate value
of 5 and we get
hc » b/6 · f. |

| Now here is a simple formula! |
|
 |
But how good is it? Well, we live in the age of easy accessible
PCs with tremendous computing power, so solving the transcendental equation from above is
actually no problem at all. The result for a suitable set of parameters is shown in the backbone part of the Hyperscript; here we only note: |
|  | The best approximation is actually obtained for ln y=3,03 leading
to |
| | |
| | |
| | |
|
 |
So if your misfit is 1% (f=0,01), your critical thickness will
be roughly around 10 b. Burger vectors usually are b=a/2<110> or b=a/21/2
which is around 0,3 nm. This gives
hc »
3 nm - which is not all that much! |
|  | Fortunately, as Sir Peter points out in the remainder of the article,
the critical thicknesses observed are usually considerably larger than the calculated ones. |