|
(Dielectric) polarization mechanisms in dielectrics are all mechanisms that
- Induce dipoles at all (always with µ in field direction)
Þ Electronic polarization.
- Induce dipoles already present in the material to "point" to some extent in field direction.
Þ Interface polarization.
Þ Ionic polarization.
Þ Orientation polarization. |
|
Quantitative considerations of polarization mechanisms yield
- Justification (and limits) to the P µ
E "law"
- Values for c
- c = c(w)
- c = c(structure)
|
|
| |
| |
 |
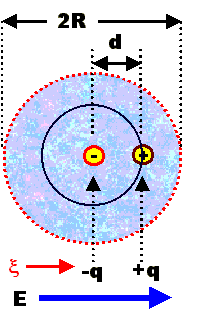
Electronic polarization describes the separation of the centers of "gravity"
of the electron charges in orbitals and the positive charge in the nucleus and the dipoles formed this way. it is always
present | |
|
|
 |
It is a very weak effect in (more or less isolated) atoms or ions with spherical
symmetry (and easily calculated). | |
|
 |
It can be a strong effect in e.g. covalently bonded materials like Si (and not so easily
calculated) or generally, in solids. | |
| | |
| |
 |
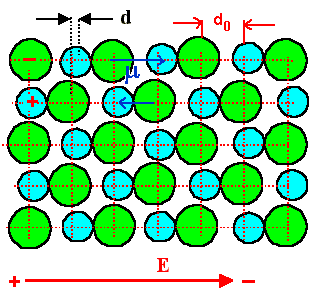
Ionic polarization describes the net effect of changing the distance between neighboring
ions in an ionic crystal like NaCl (or in crystals with some ionic component like SiO2) by the
electric field | |
|
|
 |
Polarization is linked to bonding strength, i.e. Young's modulus Y. The effect is smaller
for "stiff" materials, i.e.
P µ 1/Y |
|
| |
| |
| |
 |
Orientation polarization results from minimizing the free enthalpy of an ensemble
of (molecular) dipoles that can move and rotate freely, i.e. polar liquids. |
|
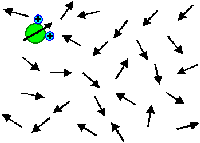 |
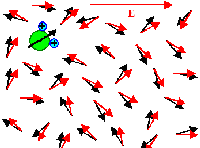 | | Without field | With field |
|
|
 |
It is possible to calculate the effect, the result invokes the Langevin function |
|
|
|
| |
|
|
|
|
 |
In a good approximation the polarization is given by Þ |
|
|
| | |
| |
|
 |
The induced dipole moment µ in all mechanisms is proportional to the
field (for reasonable field strengths) at the location of the atoms / molecules considered. |
|
|
|
 |
The proportionality constant is called polarizability a;
it is a microscopic quantity describing what atoms or molecules "do" in a field. |
|
|
 |
The local field, however, is not identical to the macroscopic or external field, but can be
obtained from this by the Lorentz approach | |
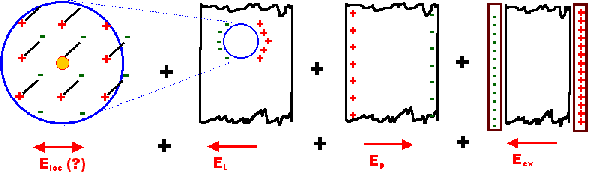 |
| Eloc = Eex + Epol
+ EL + Enear |
|
|
|
 |
For isotropic materials (e.g. cubic crystals) one obtains |
|
|
| |
|
|
|
| |
 |
Knowing the local field, it is now possible to relate the microscopic quantity
a to the macroscopic quantity e or er
via the Clausius - Mosotti equations Þ |
|
N · a
3 e0 |
= |
er – 1
er + 2 | | |
| |
| = |
c
c + 3 |
|
|
|
 |
While this is not overly important in the engineering practice, it is a momentous achievement.
With the Clausius - Mosotti equations and what went into them, it was possible for the first time to understand most electronic
and optical properties of dielectrics in terms of their constituents (= atoms) and their structure (bonding, crystal lattices
etc.) | |
|
 |
Quite a bit of the formalism used can be carried over to other systems with dipoles involved,
in particular magnetism = behavior of magnetic dipoles in magnetic fields. |
|
| |
|
|
© H. Föll (Electronic Materials - Script)