 |
The wave vector of the primary electron beam is rather large compared to typical
reciprocal lattice vectors; any small part of the the Ewald
sphere is almost a straight line or plane in 3-D, respectively. |
|
 |
For a thin foil the points in reciprocal space become elongated perpendicular to the foil;
the flat Ewald sphere the can cut through many reciprocal lattice points - many reflexes are excited! |
|
 |
In very thin specimens where inelastic scattering is negligible, the diffraction pattern then consists
of many reflexes with intensities that decrease as the excitation error increases. It is nearly impossible to establish
precise diffraction conditions; e.g. a two-beam case with a defined excitation error. |
 |
Fortunately, with a bit of inelastic scattering, electrons that are first scattered
inelastically and then elastically, form a system of lines, so-called Kikuchi
lines, which give a precise picture of the diffraction conditions. |
| |
|
|
 |
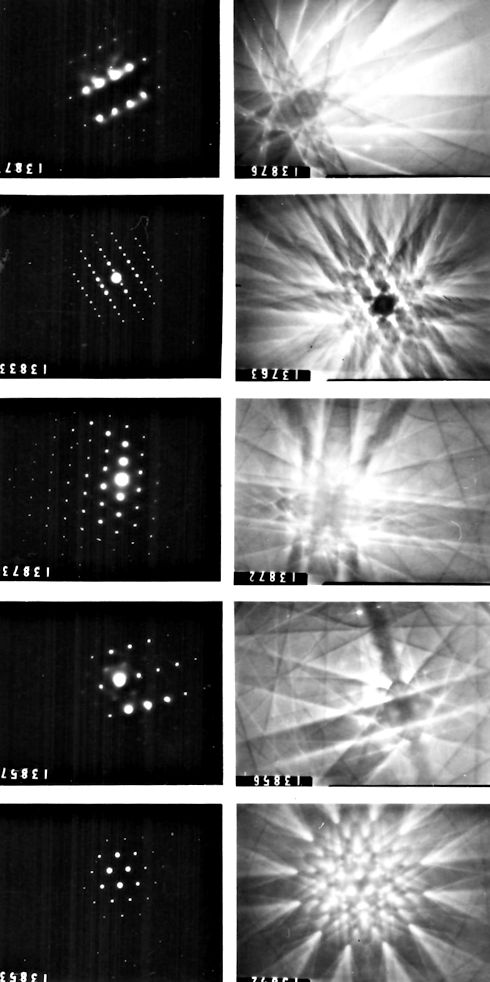
Shown are some diffraction patterns; on the left from "thick" specimens with Kikuchi lines, on
the right from "thin" cases with the same orientation and without visible Kikuchi lines. |
|
 |
So, simply move to thick part of your specimen, where with some practice and the help of a "Kikuchi
Map", it is easy to tilt the specimen to any desired orientation with high precision, and then go back to a thin part. |
| | |
© H. Föll (Defects - Script)
![]() 6.3.1 Basics of TEM and the Contrast of Dislocations
6.3.1 Basics of TEM and the Contrast of Dislocations