In section 1.2 we discussed properties of electrons and holes within an intrinsic semiconductor. In this section we will discuss mixing of other atoms into a pure solid (Si) and some consequences for the electrons and holes.
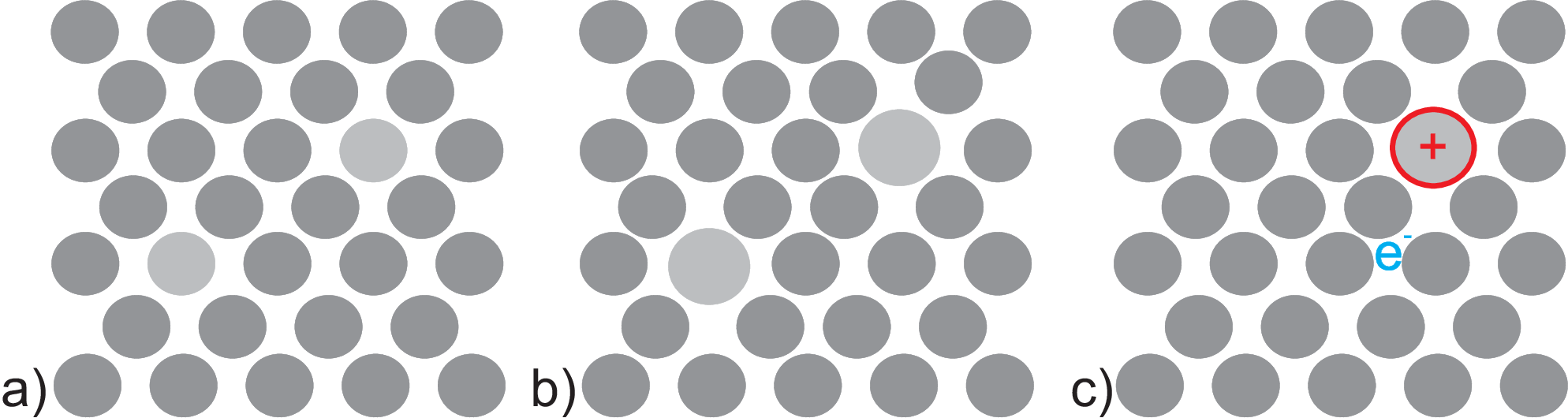
Fig. 1.2 shows schematically three mixing condition
Mixing chemically identical, e.g. different isotopes into Si
Mixing chemically different but uncharged, e.g. Ge into Si
Mixing dopants, e.g. B or P into Si
Non idealities which are not described by Eq. (1.30) can be incorporated by introducing an effective pressure (completely analogous to the concept of effective masses) called fugacity \(f\), leading to
|
| \begin{equation*} \mu = \mu^0 + R T \ln \frac{f}{p^0} \quad . \label{mu_f_1} \end{equation*} | (1.40) |
The same concepts hold for ideal and non ideal solutions (mixing of liquids) and for the
condition shown in Fig. 1.2 a). Since different
isotopes have identical chemical properties just the uncertainty, which kind of atom will occupy a position, increases,
i.e the entropy increases in thermodynamic equilibrium.
Thermodynamic equilibrium needs for the homogeneous
distribution of atoms after mixing. This nearly automatically happens when mixing gases, it often just needs for some stirring
when mixing liquids, but can be very difficult when mixing solids. As is well known just bringing ”two blocks”
of different isotopes of silicon in close connection at room temperature would never (longer than the life time of the universe)
lead to a homogeneous distribution of both isotopes. It needs for high temperatures, Brownian motion, diffusion to reach
a homogeneous distribution. In solid state physics the forces and time constants to reach thermodynamic equilibrium are
often much more important than the properties at thermodynamic equilibrium.
In Fig. 1.2 b) a mixture of silicon and germanium is shown. In addition
to the problem of generating a homogeneous mixing the much larger germanium atoms will induce an enthalpy contribution to
the mixing. To have any significant impact a molar fraction of germanium in the order of percent is necessary. Silicon atoms
will only slightly be affected by this small amount of germanium. Raoult’s law will be applicable for the partial
pressure:
|
| \begin{equation*} p_{Si} = x_{Si} p_{Si}^{\star} \quad. \end{equation*} | (1.41) |
For the partial pressure of the germanium atoms Henry’s law will hold, i.e.
|
| \begin{equation*} p_{Ge} = x_{Ge} K_{Ge} \quad. \end{equation*} | (1.42) |
The effect on electrons and holes will be much stronger and more important. Due to the
larger Ge atoms the silicon atoms will have a smaller average distance, increasing the overlap of the covalent sp\(^3\) orbitals, thus increasing the curvatures of the bands and decreasing the effective masses of holes and electrons.
In today’s CMOS devices this is one standard trick to increase the mobility of electrons and holes within the inversion
channels and thus allowing for higher CPU frequencies. Here we see a typical problem often showing up in solid state physics:
Thermodynamic equilibrium and properties extracted from it must be discussed separately for the atoms, electrons, and holes.
For gases and liquids typically charges and atoms are connected much more tightly. This is the reason why many problems
in chemistry only need for a classical approaches. In the space which an atom occupies an electron can find many different
”positions”, i.e. the number of possible quantum mechanically allowed states is typically much larger
than the number of electrons which is the prerequisite for the Boltzmann approximation.
Fig. 1.2 c) shows doping, i.e. mixing of silicon
with atoms which introduce additional ”free” charges into the electronic band structure. As is well known already
a typical doping of \(10^{16}\) cm\(^{-3}\) has a tremendous effect on the electronic properties,
i.e. a molar fraction of around \(10^{-6}\) significantly changes the position of the Fermi energy (i.e. chemical
potential \(\mu\)). Here we again switched from the effects of mixing of atoms to the effects on (free) charge
carriers (electrons and/or holes). For semiconductor devices thermodynamic equilibrium of the doping atoms is often completely
undesired, e.g. a pn-junction is the essential ingredient for ”switching” described by the diode equation.
The essence for diode behavior is the existence of a space charge region (SCR). The most simple approach
to describe a space charge region is to assume an abrupt SCR, solve the classical Poisson equation and find a self consistent
solution for the width \(W\) (typically several hundred nm) of the SCR by enforcing charge neutrality across
the interface leading to
|
| \begin{equation*} W \propto \sqrt{U_{bi}-U} \quad. \end{equation*} | (1.43) |
Exactly the same approach is used to calculate the Debye length \(d_D\) which is the charged layer at the surface of metals (typically in the order of nm). Here the approach is
|
| \begin{equation*} \exp \left(- \frac{x}{d_D} \right) \quad. \end{equation*} | (1.44) |
Essentials:
Introducing/teaching fundamental concepts of Thermodynamics and Kinetics within the framework of solid state physics is difficult (useless) because
thermodynamic equilibrium is more difficult to reach within a solid
often (for the atoms) not even desired and existing
atoms, electrons, and holes are less tightly connected
quantum mechanical aspects are more often to be taken into account
atoms, electrons, and holes have to be treated by different approximation
non idealities are described by effective parameters which show the same functionality as the parameters for the ideal case
even extremely small charge concentrations lead to strong non ideal behavior
charged interfaces are calculated by the (classical) Poisson equation using a self consistent approach to guarantee charge neutrality across the interface
© J. Carstensen (TD Kin II)