|
The dielectric constant er "somehow"
describes the interaction of dielectric (i.e. more or less insulating) materials and electrical fields; e.g. via the equations
Þ | |
|
|
 |
D is the electrical displacement or electrical
flux density, sort of replacing E in the Maxwell equations whenever materials
are encountered. | |
|
 |
C is the capacity of a parallel plate capacitor (plate area A,
distance d) that is "filled" with a dielectric with er |
|
|
 |
n is the index of refraction; a quantity that "somehow" describes
how electromagnetic fields with extremely high frequency interact with matter.
in this equaiton it is assumed that the
material has no magnetic properties at the frequency of light. |
|
| |
| |
| |
 |
Electrical fields inside dielectrics polarize the material, meaning that the vector
sum of electrical dipoles inside the material is no longer zero. |
| |
|
 |
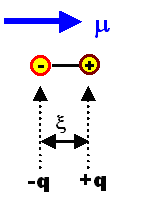
The decisive quantities are the dipole moment µ, a vector, and the Polarization
P, a vector, too. | |
|
|
 |
Note: The dipole moment vector points from the negative to the positive charge - contrary
to the electrical field vector! | |
|
 |
The dipoles to be polarized are either already present in the material (e.g. in H2O
or in ionic crystals) or are induced by the electrical field (e.g. in single atoms or covalently bonded crystals like Si) |
|
|
 |
The dimension of the polarization P is [C/cm2] and
is indeed identical to the net charge found on unit area ion the surface of a polarized dielectric. |
|
| |
| |
| |
 |
The equivalent of "Ohm's law", linking current density to field strength
in conductors is the Polarization law: | |
|
|
 |
The decisive material parameter is c ("kee"), the
dielectric susceptibility |
|
|
 |
The "classical" flux density D and the Polarization are linked as
shown. In essence, P only considers what happens in the material, while D looks at the
total effect: material plus the field that induces the polarization. |
|
| |
| |
|
 |
Polarization by necessity moves masses (electrons and / or atoms) around, this
will not happen arbitrarily fast. | |
|
|
 |
er or c thus
must be functions of the frequency of the applied electrical field, and we want to consider the whole frequency range from
RF via HF to light and beyond. | |
| er(w) is called the "dielectric
function" of the material. |
|
 |
The tasks are:
- Identify and (quantitatively) describe the major mechanisms of polarization.
- Justify the assumed linear relationship between P and c.
- Derive the dielectric function for a given material.
|
| |
| | |
|
© H. Föll (Electronic Materials - Script)