Solution to Exercise 2.1-3"Calculate the Formation Entropy"

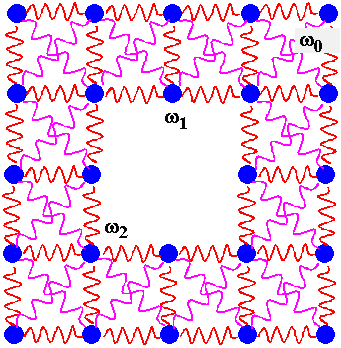
	Lets look at a simple cubic lattice containing one vacancy and connect the atoms by springs symbolizing the bonds. It looks like this:
				We have two kinds of springs: The red ones connect nearest neighbors and will heavily influence the vibration frequencies. The violet ones, connecting diagonally. They will have some bearing on the vibration frequency, but since they must be weaker (the bond is weaker) than the red springs, their influence should be less pronounced.
				However, without the violet springs you could not make a stable crystal if you tried to built a model with balls and springs.
				Lets assign a spring constant D to the red springs and c · D to the violet springs, with c < 1, and see what we get for the vibration frequency of an atom completely surrounded by other atoms and for the atoms around the vacancy.

	Generally, the resonance (circular) frequency w of a particle with mass m is given by
		w2  =  D m
	In the most simple approximation, only accounting for the red springs, a regular atom would feel the force of two springs per direction and thus vibrate in any of the three dimensions with
		.
		w02  =  2D m
	The six atoms (for three dimensions) surrounding the vacancy and missing one red spring each (in one dimension), in contrast, would vibrate in one of the three dimensions with
		w12  =  D m
	The entropy of formation SF then becomes (note that we only have to sum over the "afflicted" dimensions):
		SF  =  k ·  6 S  1   ln w0 w1   =  k · 6 · ln (2)1/2  =  3k · ln 2 = 3k · 0,693                  =  2,08 k
	Not bad for such a simple approximation. But now lets go one step further and add the violet springs.
		We have now for the frequency of the lattice atoms without a vacancy
		w02  =  2D  +  4c D m
		and we simply include the factor (1/2)–1/2, that would give us the component of the violet springs in the direction considered, into c; we thus have c < 0,707.
	We now have to consider the 6 atoms with a missing red spring and 2 missing violet springs separately from the 12 atoms just missing one violet spring which are vibrating with w2, and consider the changed w0, too. Altogether we have
		w02  =  2D  +  4cD m       w12  =  D   + 2cD m       w22  =  2D  +  3cD m
	The entropy now is
		SF  = k · æ ç è  6 S  1   ln w0 w1   +   12 S  1   ln w0 w2 ö ÷ ø
		Crunching the numbers gives
		SF  =  3k · ln 2 + 4c 1 + 2c  +  6 · ln 2 + 4c 2 + 3c  =  3k ·ln (2) + 6k · ln  2 + 4c 2 + 3c
		For c = 0 we must obtain our old result which indeed we do (check it), and for c = 0,707, the most extreme case possible, we find
		SF  =  3k · ln (2) + 6k · ln (1,171)  =  2,08 k  +  0,947k  =  3,027 k
	In other words: For realistic c values, the correction is negligible and we can confidently claim that the formation entropy of a monovacancy in a cubic primitive lattice is around 2 k in our ball and spring model approximation.

With frame

Ex. 2.1-3 Formation Entropy

© H. Föll (Defects - Script)